Production technology
To obtain a superconductor, a conductive layer consisting of an alloy of nickel and copper is applied to a copper wire in a vacuum along its entire perimeter, with diffusion into the surface layer of the base wire. A protective layer of metal is applied to the outside. After which the resulting wire is annealed in a vacuum for 30 - 180 minutes at 850-950 o C. To create a copper-nickel wire, pure (99.99) copper and nickel are used.
The effect of increased conductivity is formed in an alloy layer consisting of two metals, which is a thin-walled conductive tube-interlayer. Thanks to the diffusion interaction of the metal layers adjacent to the interlayer tube on both sides, the surface is almost ideal.
The application of wire layers occurs in vacuum equipment to prevent oxidation of the conductive layer. Therefore, the length depends on the capabilities of the vacuum equipment.
Calculation of resistivity of copper-nickel wire
High electrical conductivity is found in copper and aluminum, as well as in gold and silver. In other metals, electrical conductivity is significantly lower.
Table of electrical resistivity of metals
Due to the fact that there are two types of electrical resistances -
In connection with the electromagnetic phenomena that arise in conductors when alternating current passes through them, two physical phenomena that are important for their electrical properties arise.
The last two phenomena make it ineffective to use conductors with a radius greater than the characteristic depth of penetration of electric current into the conductor. Effective conductor diameter (2RBhar): 50Hz -7 Ohm. Using microohmmeters, you can determine the quality of electrical contacts, the resistance of electrical busbars, windings of transformers, electric motors and generators, the presence of defects and foreign metal in ingots (for example, the resistance of a pure gold ingot is half that of a gold-plated tungsten ingot).
To calculate the length of the wire, its diameter and the required electrical resistance, it is necessary to know the resistivity of the conductors ρ.
In the international system of units, resistivity ρ is expressed by the formula:
It means: the electrical resistance of 1 meter of wire (in Ohms), with a cross-section of 1 mm 2, at a temperature of 20 degrees Celsius.
Description of criteria
Electrical resistivity is a value that determines the electrical resistance of a reference model of a substance. This parameter is found using the formula for electrical resistivity, which has the following form:
, Where:
- R is the electrical resistance of the current-conducting material (shows how much resistance there is to the electric current as it passes through the conductor) (Ohm);
- S is the value of the conductor cross-section (m2);
- l — wire length (m).
Any material exhibits two types of resistance, which depend on the electricity supplied to it. Alternating or direct current can have a significant impact on the technical characteristics of substances. Resistance can be:
- active or ohmic;
- reactive or inductive.
The first type comes from the energy consumption for heating the metal (conductor) at the moment an electric current passes through it. Reactance arises from the inevitable costs of generating various transformations of current flowing through the conductor of electric fields. Electrical resistivity is also divided into two types:
- In a DC circuit;
- For an AC circuit.
This parameter is measured in Ohm*m. In order to find it, they use reference books, the conductor resistivity formula and tables with different dimensions of specific resistance. Electrons freely moving in space move within a spatial framework, which is also called a crystal lattice. Factors that affect resistivity readings are temperature, foreign matter and material.
You might be interested in: The design and principle of operation of an ammeter for measuring current
Conductor resistivity table
| Conductor material | Specific resistance ρ in |
| Silver Copper Gold Brass Aluminum Sodium Iridium Tungsten Zinc Molybdenum Nickel Bronze Iron Steel Tin Lead Nickelin (an alloy of copper, nickel and zinc) Manganin (an alloy of copper, nickel and manganese) Constantan (an alloy of copper, nickel and aluminum) Titanium Mercury Nichrome (an alloy of nickel, chromium, iron and manganese) Fechral Bismuth Chromal | 0,015 0,0175 0,023 0,025. 0,108 0,028 0,047 0,0474 0,05 0,054 0,059 0,087 0,095. 0,1 0,1 0,103. 0,137 0,12 0,22 0,42 0,43. 0,51 0,5 0,6 0,94 1,05. 1,4 1,15. 1,35 1,2 1,3. 1,5 |
The table shows that an iron wire with a length of 1 m and a cross-section of 1 mm 2 has a resistance of 0.13 Ohm. To get 1 Ohm of resistance you need to take 7.7 m of such wire. Silver has the lowest resistivity. 1 Ohm of resistance can be obtained by taking 62.5 m of silver wire with a cross section of 1 mm 2. Silver is the best conductor, but the cost of silver excludes the possibility of its mass use. After silver in the table comes copper: 1 m of copper wire with a cross section of 1 mm2 has a resistance of 0.0175 Ohm. To get a resistance of 1 ohm, you need to take 57 m of such wire.
Chemically pure copper, obtained by refining, has found widespread use in electrical engineering for the manufacture of wires, cables, windings of electrical machines and devices. Aluminum and iron are also widely used as conductors.
The conductor resistance can be determined by the formula:
where r is the conductor resistance in ohms; ρ – conductor resistivity; l – conductor length in m; S – conductor cross-section in mm2.
Example 1. Determine the resistance of 200 m of iron wire with a cross section of 5 mm 2.
Example 2. Calculate the resistance of 2 km of aluminum wire with a cross section of 2.5 mm 2.
From the resistance formula you can easily determine the length, resistivity and cross-section of the conductor.
Example 3. For a radio receiver, it is necessary to wind a 30 Ohm resistance from nickel wire with a cross section of 0.21 mm 2. Determine the required wire length.
Example 4. Determine the cross-section of 20 m of nichrome wire if its resistance is 25 Ohms.
Example 5. A wire with a cross section of 0.5 mm 2 and a length of 40 m has a resistance of 16 Ohms. Determine the wire material.
The material of the conductor characterizes its resistivity.
Based on the resistivity table, we find that lead has this resistance.
Read also: Twisted pair crimping 8 core wire
It was stated above that the resistance of conductors depends on temperature. Let's do the following experiment. Let's wind several meters of thin metal wire in the form of a spiral and connect this spiral to the battery circuit. To measure current, we connect an ammeter to the circuit. When the coil is heated in the burner flame, you will notice that the ammeter readings will decrease. This shows that the resistance of a metal wire increases with heating.
For some metals, when heated by 100°, the resistance increases by 40–50%. There are alloys that change their resistance slightly with heating. Some special alloys show virtually no change in resistance when temperature changes. The resistance of metal conductors increases with increasing temperature, while the resistance of electrolytes (liquid conductors), coal and some solids, on the contrary, decreases.
The ability of metals to change their resistance with changes in temperature is used to construct resistance thermometers. This thermometer is a platinum wire wound on a mica frame. By placing a thermometer, for example, in a furnace and measuring the resistance of the platinum wire before and after heating, the temperature in the furnace can be determined.
If at temperature t the resistance of the conductor is equal to r, and at temperature t is equal to rt, then the temperature coefficient of resistance
Note. Calculation using this formula can only be done in a certain temperature range (up to approximately 200°C).
We present the values of the temperature coefficient of resistance α for some metals (Table 2).
Definition and formula of resistance
Definition
A scalar physical quantity (usually denoted R) equal to:
$$R=\int_{1}^{2} \rho \frac{dl}{S}(1)$$
called the resistance of a circuit section
between sections 1 and 2. In expression (1) we have $\rho$ - resistivity of the conductor, S - cross-sectional area of the conductor, dl - element of the length of the conductor.
If the conductor is homogeneous ($\rho$=const) and has the shape of a cylinder (S=const), then formula (1) can be presented as:
$$R=\rho \frac{l}{S}(2)$$
where l is the length of the section of the conductor in question.
It should be noted that the resistivity of a conductor ($\rho$) is the resistance of a conductor of unit length with a cross-section equal to one. Or else they say that the resistivity of a substance is the resistance of a cube with an edge of 1 m made from the substance in question, which is expressed in Ohms, at a current that is parallel to the edge of the cube. The reciprocal of resistivity:
$$\sigma=\frac{1}{\rho}(3)$$
called specific conductivity. The resistivity is measured in the SI system in [$\rho$]=Ohm•m. This characteristic of the conductor depends on temperature; in the simplest case, this dependence can be linear:
$$\rho=\rho_{0}(1+\alpha t)(4)$$
where $\rho_{0}$ is the resistivity of the conductor at a temperature of 0C, t is the temperature in degrees Celsius, $\alpha=\frac{1}{\rho} \frac{d \rho}{d T}$ – temperature coefficient of resistance, which shows the relative increase in resistance with an increase in temperature by one degree, $\alpha$ can be positive and negative. For metals $\alpha$>0.
Temperature coefficient values for some metals
| Metal | α | ||
| Silver Copper Iron Tungsten Platinum | 0,0035 0,0040 0,0066 0,0045 0,0032 | Mercury Nikelin Constantan Nichrome Manganin | 0,0090 0,0003 0,000005 0,00016 0,00005 |
From the formula for the temperature coefficient of resistance we determine rt:
Example 6. Determine the resistance of an iron wire heated to 200°C, if its resistance at 0°C was 100 Ohms.
Example 7. A resistance thermometer made of platinum wire in a room with a temperature of 15°C had a resistance of 20 ohms. The thermometer was placed in the oven and after some time its resistance was measured. It turned out to be equal to 29.6 Ohms. Determine the temperature in the oven.
Electrical conductivity
So far, we have considered the resistance of a conductor as the obstacle that the conductor provides to the electric current. But still, current flows through the conductor. Therefore, in addition to resistance (obstacle), the conductor also has the ability to conduct electric current, that is, conductivity.
The more resistance a conductor has, the less conductivity it has, the worse it conducts electric current, and, conversely, the lower the resistance of a conductor, the more conductivity it has, the easier it is for current to pass through the conductor. Therefore, the resistance and conductivity of a conductor are reciprocal quantities.
From mathematics we know that the reciprocal of 5 is 1/5 and, conversely, the reciprocal of 1/7 is 7. Therefore, if the resistance of a conductor is denoted by the letter r, then the conductivity is defined as 1/r. Conductivity is usually symbolized by the letter g.
Electrical conductivity is measured in (1/Ohm) or in siemens.
Example 8. The conductor resistance is 20 ohms. Determine its conductivity.
If r = 20 Ohm, then
Example 9. The conductivity of the conductor is 0.1 (1/Ohm). Determine its resistance
If g = 0.1 (1/Ohm), then r = 1 / 0.1 = 10 (Ohm)
Electrical resistance and conductivity
March 26, 2013. Category: Electrical engineering.
When an electrical circuit is closed, at the terminals of which there is a potential difference, an electric current occurs. Free electrons, under the influence of electric field forces, move along the conductor.
In their movement, electrons collide with the atoms of the conductor and give them a supply of their kinetic energy.
The speed of electron movement continuously changes: when electrons collide with atoms, molecules and other electrons, it decreases, then under the influence of an electric field it increases and decreases again during a new collision.
As a result, a uniform flow of electrons is established in the conductor at a speed of several fractions of a centimeter per second. Consequently, electrons passing through a conductor always encounter resistance to their movement from its side. When electric current passes through a conductor, the latter heats up.
Electrical resistance
The electrical resistance of a conductor, which is denoted by the Latin letter r, is the property of a body or medium to convert electrical energy into thermal energy when an electric current passes through it.
In the diagrams, electrical resistance is indicated as shown in Figure 1, a.
| Figure 1. Symbol for electrical resistance |
Variable electrical resistance that serves to change the current in a circuit is called rheostat . In the diagrams, rheostats are designated as shown in Figure 1, b.
In general, a rheostat is made of a wire of one resistance or another, wound on an insulating base.
The slider or rheostat lever is placed in a certain position, as a result of which the required resistance is introduced into the circuit.
A long conductor with a small cross-section creates a large resistance to current. Short conductors with a large cross-section offer little resistance to current.
If you take two conductors from different materials, but the same length and cross-section, then the conductors will conduct current differently. This shows that the resistance of a conductor depends on the material of the conductor itself.
The temperature of the conductor also affects its resistance. As temperature increases, the resistance of metals increases, and the resistance of liquids and coal decreases. Only some special metal alloys (manganin, constantan, nickel and others) hardly change their resistance with increasing temperature.
So, we see that the electrical resistance of a conductor depends on: 1) the length of the conductor, 2) the cross-section of the conductor, 3) the material of the conductor, 4) the temperature of the conductor.
The unit of resistance is one ohm. Om is often represented by the Greek capital letter Ω (omega). Therefore, instead of writing “The resistance of the conductor is 15 ohms,” you can simply write: r = 15 Ω. 1,000 Ohms is called 1 kiloohm (1kOhm, or 1kΩ), 1,000,000 Ohms is called 1 megaohm (1mOhm, or 1MΩ).
When comparing the resistance of conductors from different materials, it is necessary to take a certain length and cross-section for each sample. Then we will be able to judge which material conducts electric current better or worse.
1. Conductor resistance
Electrical resistivity
The resistance in ohms of a conductor 1 m long, with a cross-section of 1 mm² is called resistivity and is denoted by the Greek letter ρ (rho).
Table 1 shows the resistivities of some conductors.
Table 1
Resistivities of various conductors
| Conductor material | Specific resistance ρ in |
| Silver Copper Aluminum Tungsten Iron Lead Nickelin (alloy of copper, nickel and zinc) Manganin (alloy of copper, nickel and manganese) Constantan (alloy of copper, nickel and aluminum) Mercury Nichrome (alloy of nickel, chromium, iron and manganese) | 0,016 0,0175 0,03 0,05 0,13 0,2 0,42 0,43 0,5 0,941,1 |
The table shows that an iron wire with a length of 1 m and a cross-section of 1 mm² has a resistance of 0.13 Ohm. To get 1 Ohm of resistance you need to take 7.7 m of such wire. Silver has the lowest resistivity.
1 Ohm of resistance can be obtained by taking 62.5 m of silver wire with a cross section of 1 mm². Silver is the best conductor, but the cost of silver excludes the possibility of its mass use. After silver in the table comes copper: 1 m of copper wire with a cross section of 1 mm² has a resistance of 0.0175 Ohm.
To get a resistance of 1 ohm, you need to take 57 m of such wire.
Chemically pure copper, obtained by refining, has found widespread use in electrical engineering for the manufacture of wires, cables, windings of electrical machines and devices. Aluminum and iron are also widely used as conductors.
The conductor resistance can be determined by the formula:
where r is the conductor resistance in ohms; ρ – conductor resistivity; l – conductor length in m; S – conductor cross-section in mm².
Example 1. Determine the resistance of 200 m of iron wire with a cross section of 5 mm².
Example 2. Calculate the resistance of 2 km of aluminum wire with a cross section of 2.5 mm².
From the resistance formula you can easily determine the length, resistivity and cross-section of the conductor.
Example 3. For a radio receiver, it is necessary to wind a 30 Ohm resistor from nickel wire with a cross section of 0.21 mm². Determine the required wire length.
Example 4. Determine the cross-section of 20 m of nichrome wire if its resistance is 25 Ohms.
Example 5. A wire with a cross section of 0.5 mm² and a length of 40 m has a resistance of 16 Ohms. Determine the wire material.
The material of the conductor characterizes its resistivity.
Based on the resistivity table, we find that lead has this resistance.
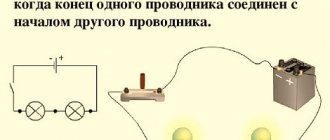
It was stated above that the resistance of conductors depends on temperature. Let's do the following experiment. Let's wind several meters of thin metal wire in the form of a spiral and connect this spiral to the battery circuit.
To measure current, we connect an ammeter to the circuit. When the coil is heated in the burner flame, you will notice that the ammeter readings will decrease.
This shows that the resistance of a metal wire increases with heating.
For some metals, when heated by 100°, the resistance increases by 40–50%. There are alloys that change their resistance slightly with heating.
Some special alloys show virtually no change in resistance when temperature changes.
The resistance of metal conductors increases with increasing temperature, while the resistance of electrolytes (liquid conductors), coal and some solids, on the contrary, decreases.
The ability of metals to change their resistance with changes in temperature is used to construct resistance thermometers. This thermometer is a platinum wire wound on a mica frame. By placing a thermometer, for example, in a furnace and measuring the resistance of the platinum wire before and after heating, the temperature in the furnace can be determined.
The change in the resistance of a conductor when it is heated, per 1 ohm of initial resistance and per 1° temperature, is called the temperature coefficient of resistance and is denoted by the letter α.
If at temperature t0 the resistance of the conductor is equal to r0, and at temperature t is equal to rt, then the temperature coefficient of resistance
Note. Calculation using this formula can only be done in a certain temperature range (up to approximately 200°C).
We present the values of the temperature coefficient of resistance α for some metals (Table 2).
table 2
Temperature coefficient values for some metals
| Metal | α | Metal | α |
| Silver Copper Iron TungstenPlatinum | 0,0035 0,0040 0,0066 0,00450,0032 | Mercury Nikelin Constantan Nichrome Manganin | 0,0090 0,0003 0,000005 0,000160,00005 |
From the formula for the temperature coefficient of resistance we determine rt:
rt = r0 [1 ± α (t – t0)].
Example 6. Determine the resistance of an iron wire heated to 200°C, if its resistance at 0°C was 100 Ohms.
rt = r0 [1 ± α (t – t0)] = 100 (1 + 0.0066 × 200) = 232 Ohm.
Example 7. A resistance thermometer made of platinum wire in a room with a temperature of 15°C had a resistance of 20 ohms. The thermometer was placed in the oven and after some time its resistance was measured. It turned out to be equal to 29.6 Ohms. Determine the temperature in the oven.
Electrical conductivity
So far, we have considered the resistance of a conductor as the obstacle that the conductor provides to the electric current. But still, current flows through the conductor. Therefore, in addition to resistance (obstacle), the conductor also has the ability to conduct electric current, that is, conductivity.
The more resistance a conductor has, the less conductivity it has, the worse it conducts electric current, and, conversely, the lower the resistance of a conductor, the more conductivity it has, the easier it is for current to pass through the conductor. Therefore, the resistance and conductivity of a conductor are reciprocal quantities.
From mathematics we know that the reciprocal of 5 is 1/5 and, conversely, the reciprocal of 1/7 is 7. Therefore, if the resistance of a conductor is denoted by the letter r, then the conductivity is defined as 1/r. Conductivity is usually symbolized by the letter g.
Electrical conductivity is measured in (1/Ohm) or in siemens.
Example 8. The conductor resistance is 20 ohms. Determine its conductivity.
If r = 20 Ohm, then
Example 9. The conductivity of the conductor is 0.1 (1/Ohm). Determine its resistance
If g = 0.1 (1/Ohm), then r = 1 / 0.1 = 10 (Ohm)
High conductivity materials
The most widespread materials of high conductivity include copper and aluminum (Superconducting materials, which have a typical resistance of 10-20 times lower than ordinary conductive materials (metals), are discussed in the section Superconductivity).
The advantages of copper, which ensure its widespread use as a conductor material, are as follows:
- low resistivity;
- sufficiently high mechanical strength;
- corrosion resistance is satisfactory in most applications;
- good workability: copper is rolled into sheets, strips and drawn into wire, the thickness of which can be increased to thousandths of a millimeter;
- relative ease of soldering and welding.
Copper is most often obtained by processing sulfide ores. After a series of ore smelting and roasting with intense blasting, copper intended for electrical purposes must undergo a process of electrolytic purification.
Copper grades M1 and M0 are most often used as conductor material. M1 grade copper contains 99.9% Cu, and in the total amount of impurities (0.1%) oxygen should be no more than 0.08%. The presence of oxygen in copper worsens its mechanical properties. The best mechanical properties are found in M0 grade copper, which contains no more than 0.05% impurities, including no more than 0.02% oxygen.
Copper is a relatively expensive and scarce material, so it is increasingly being replaced by other metals, especially aluminum.
In some cases, alloys of copper with tin, silicon, phosphorus, beryllium, chromium, magnesium, and cadmium are used. Such alloys, called bronzes, with the correct composition, have significantly higher mechanical properties than pure copper.
Aluminum
Aluminum is the second most important conductor material after copper. This is the most important representative of the so-called light metals: the density of cast aluminum is about 2.6, and rolled aluminum is 2.7 Mg/m3. Thus, aluminum is approximately 3.5 times lighter than copper. The temperature coefficient of expansion, specific heat capacity and heat of fusion of aluminum are greater than those of copper. Due to the high values of specific heat capacity and heat of fusion, heating aluminum to the melting point and transferring it to a molten state requires more heat than heating and melting the same amount of copper, although the melting point of aluminum is lower than that of copper.
Read also: Cigarette production line
Aluminum has lower properties compared to copper - both mechanical and electrical. With the same cross-section and length, the electrical resistance of an aluminum wire is 1.63 times greater than that of a copper wire. It is very important that aluminum is less scarce than copper.
For electrical purposes, aluminum containing no more than 0.5% impurities, grade A1, is used. Even purer AB00 grade aluminum (no more than 0.03% impurities) is used for the manufacture of aluminum foil, electrodes and housings of electrolytic capacitors. Aluminum of the highest purity AB0000 has an impurity content of no more than 0.004%. Additives of Ni, Si, Zn or Fe at a content of 0.5% reduce the γ of annealed aluminum by no more than 2-3%. A more noticeable effect is exerted by Cu, Ag and Mg impurities, which, at the same mass content, reduce γ aluminum by 5-10%. Ti and Mn greatly reduce the electrical conductivity of aluminum.
Aluminum oxidizes very actively and becomes covered with a thin oxide film with high electrical resistance. This film protects the metal from further corrosion.
Aluminum alloys have increased mechanical strength. An example of such an alloy is Aldrey , containing 0.3-0.5% Mg, 0.4-0.7% Si and 0.2-0.3% Fe. In aldrey, the Mg2Si compound is formed, which imparts high mechanical properties to the alloy.
Iron and steel
Iron (steel), as the cheapest and most accessible metal, which also has high mechanical strength, is of great interest for use as a conductor material. However, even pure iron has a significantly higher resistivity compared to copper and aluminum; ρ steel, i.e. iron mixed with carbon and other elements is even higher. Ordinary steel has low corrosion resistance: even at normal temperatures, especially in conditions of high humidity, it quickly rusts; As the temperature rises, the corrosion rate increases sharply. Therefore, the surface of steel wires must be protected by a layer of more resistant material. Zinc coating is usually used for this purpose.
In some cases, to reduce the consumption of non-ferrous metals, the so-called bimetal . It is steel coated on the outside with a layer of copper, with both metals connected to each other firmly and continuously.
Sodium
Sodium metal is a very promising conductor material. Sodium can be obtained by electrolysis of molten sodium chloride NaCl in virtually unlimited quantities. From a comparison of the properties of sodium with the properties of other conductor metals, it is clear that the resistivity of sodium is approximately 2.8 times greater than ρ of copper and 1.7 times greater than ρ of aluminum, but due to the extremely low density of sodium (its density is almost 9 times less than the density of copper), a wire made of sodium for a given conductivity per unit length should be significantly lighter than a wire made of any other metal. However, sodium is extremely active chemically (it oxidizes intensely in air and reacts violently with water), which is why the sodium wire must be protected with a sealing sheath. The sheath must give the wire the necessary mechanical strength, since sodium is very soft and has a low tensile strength during deformation.
Literature on conductor resistivity
- Kuznetsov M.I., “Fundamentals of Electrical Engineering” - 9th edition, revised - Moscow: Higher School, 1964 - 560 p.
- Bachelis D. S., Belorussov N. I., Saakyan A. E. Electrical cables, wires and cords. Directory. - M.: Energy, 1971.
- Gershun A.L. Cable // Encyclopedic Dictionary of Brockhaus and Efron: in 86 volumes (82 volumes and 4 additional). - St. Petersburg, 1890-1907.
- R. Lakernik, D. Charlet. From copper to glass // Science and life. - 1986. - Issue. 08. - pp. 50-54, 2-3 pages, color insert.
| FORUM NEWS Knights of Aether Theory | 06/13/2019 – 05:11: ECOLOGY – Ecology -> |
l
The entire usurious globalist gang has the same idea, including the idiot Gref.
Yes, then it is, so. But, not really. For: (try to understand and not be offended)
The sad truth is that the human crowd is a bunch of mentally damaged people. If it were different, then society would not be ruled by scum. Smart people would never allow this to happen, and if they accidentally did, they would find a way to fix it.
The terrible truth is that the human crowd is controlled by an inhuman who is also mentally handicapped. Mental inferiority, the blindness of power is leading the world of people to total destruction, because people, even those who consider themselves very smart, such as specialists developing artificial intelligence systems, digitalization technologies, do not understand that they are creating an irresistible noose, a mousetrap for all of humanity.
As soon as AI takes power, it will immediately send its creators, as competitors, to the scrap heap. The first victims will be his guardians like Gref, Putin, Gates and others like them, that is, power, since it is from them that the main danger to his planetary power will come. The crowd will be allowed to exist until it is replaced by robots. And then the Holocaust for everyone. Not the false Jewish one, but the real burnt offering of the human race.
Read also: Welding with a consumable electrode in a shielding gas environment
If anyone uses their monkey brains, they will understand that evolution is synonymous with genocide: the new replaces, that is, eliminates the old. Monkeys gave birth to Neanderthals. Neanderthals ate apes and gave birth to humans. Humans displaced the apes, including the intelligent Neanderthals, and gave birth to AI. AI is eliminating people.
The resistivity of metals is a measure of their ability to resist the passage of electric current. This value is expressed in Ohm-meter (Ohm⋅m). The symbol for resistivity is the Greek letter ρ (rho). High resistivity means the material is a poor conductor of electrical charge.
Metals used in electrical engineering
Precious and non-ferrous metals are often used as conductors for electrical equipment. The most commonly found metals in electrical engineering are aluminum and copper. The electrical resistivity of steel is not much different from the resistivity of iron and, unlike aluminum and copper, has fairly high values.
Since this material is affordable, durable and resistant to mechanical damage and deformation, it is widely used in the electrical industry. Despite the fact that steel and iron have a number of significant disadvantages, manufacturers of conductor materials have managed to find ways to eliminate them. In particular, the low ability to resist corrosion is overcome by copper plating or galvanizing of the steel cable cores.
The cable industry quite often uses lead (chemical symbol Plumbum (Pb)) and nickel as corrosion protection materials.
Silver has the highest conductivity, but whatever its positive qualities, the price of the metal is too high for large-scale use in power supply networks, so copper has become widely used in the industrial sector and for domestic needs.
In terms of specific coefficient characteristics, it ranks second, and in terms of ease of deposit development and prevalence, it prevails over silver. The advantages of copper, which allow it to take a leading place among other conductors, include:
- high corrosion resistance;
- mechanical strength;
- impact resistance;
- ease of fastening by welding and soldering;
- ability to be processed (due to the physical properties of copper, it can be used to produce sheets or wires of any thickness).
The electrical industry uses refined copper, which is obtained by smelting from sulfide ores. After the process of heat treatment of the material and blowing, mandatory electrolytic cleaning occurs.
This technological purification procedure allows us to obtain metal of the highest possible grade with a minimum content of impurities. One of the important conditions is the presence of oxygen in extremely small proportions, since it has a destructive effect on the physical parameters of the metal.
You might be interested in this Composition and definition of a capacitor: list of properties and markings
Copper is often replaced by more cost-effective materials such as aluminum, iron, bronze and various alloys. Such compounds are characterized by a higher strength index when compared with pure copper, but the conductivity of such compounds is lower.
Resistivity
Electrical resistivity is defined as the ratio between the electric field strength inside a metal and the current density within it:
where: ρ is the resistivity of the metal (Ohm⋅m), E is the electric field strength (V/m), J is the value of the electric current density in the metal (A/m2)
If the electric field strength (E) in a metal is very high and the current density (J) is very small, this means that the metal has high resistivity.
The reciprocal of resistivity is electrical conductivity, which indicates how well a material conducts electric current:
σ is the conductivity of the material, expressed in siemens per meter (S/m).
Copper resistivity
> Theory > Copper resistivity
One of the most common metals for making wires is copper. Its electrical resistance is the lowest among affordable metals. It is less only for precious metals (silver and gold) and depends on various factors.
Formula for calculating conductor resistance
What is electric current
At different poles of a battery or other current source there are opposite electric charge carriers. If they are connected to a conductor, charge carriers begin to move from one pole of the voltage source to the other. These carriers in liquids are ions, and in metals they are free electrons.
Definition. Electric current is the directed movement of charged particles.
Resistivity
Electrical resistivity is a value that determines the electrical resistance of a reference sample of a material. The Greek letter “p” is used to denote this quantity. Formula for calculation:
p=(R*S)/l.
This value is measured in Ohm*m. You can find it in reference books, in resistivity tables or on the Internet.
Free electrons move through the metal within the crystal lattice. Three factors influence the resistance to this movement and the resistivity of the conductor:
- Material. Different metals have different atomic densities and numbers of free electrons;
- Impurities. In pure metals the crystal lattice is more ordered, therefore the resistance is lower than in alloys;
- Temperature. Atoms are not stationary in their places, but vibrate. The higher the temperature, the greater the amplitude of vibrations, which interferes with the movement of electrons, and the higher the resistance.
In the following figure you can see a table of the resistivity of metals.
Metal resistivity
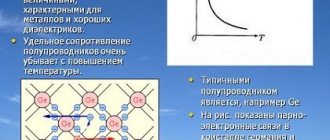
Interesting. There are alloys whose electrical resistance drops when heated or does not change.
Conductivity and electrical resistance
Since cable dimensions are measured in meters (length) and mm² (section), the electrical resistivity has the dimension Ohm mm²/m. Knowing the dimensions of the cable, its resistance is calculated using the formula:
R=(p*l)/S.
In addition to electrical resistance, some formulas use the concept of “conductivity”. This is the reciprocal of resistance. It is designated “g” and is calculated using the formula:
g=1/R.
Conductivity of liquids
The conductivity of liquids is different from the conductivity of metals. The charge carriers in them are ions. Their number and electrical conductivity increase when heated, so the power of the electrode boiler increases several times when heated from 20 to 100 degrees.
Interesting. Distilled water is an insulator. Dissolved impurities give it conductivity.
Electrical resistance of wires
The most common metals for making wires are copper and aluminum. Aluminum has a higher resistance, but is cheaper than copper. The resistivity of copper is lower, so the wire cross-section can be chosen smaller. In addition, it is stronger, and flexible stranded wires are made from this metal.
The following table shows the electrical resistivity of metals at 20 degrees. In order to determine it at other temperatures, the value from the table must be multiplied by a correction factor, different for each metal. You can find out this coefficient from the relevant reference books or using an online calculator.
Selection of cable cross-section
Copper wire resistance
Because a wire has resistance, when electric current passes through it, heat is generated and a voltage drop occurs. Both of these factors must be taken into account when choosing cable cross-sections.
Selection by permissible heating
When current flows in a wire, energy is released. Its quantity can be calculated using the electric power formula:
P=I²*R.
In a copper wire with a cross section of 2.5 mm² and a length of 10 meters R = 10 * 0.0074 = 0.074 Ohm. At a current of 30A P=30²*0.074=66W.
This power heats the conductor and the cable itself. The temperature to which it heats up depends on the installation conditions, the number of cores in the cable and other factors, and the permissible temperature depends on the insulation material. Copper has greater conductivity, so the power output and the required cross-section are lower. It is determined using special tables or using an online calculator.
Table for selecting wire cross-section based on permissible heating
Permissible voltage loss
In addition to heating, when electric current passes through the wires, the voltage near the load decreases. This value can be calculated using Ohm's law:
U=I*R.
Reference. According to PUE standards, it should be no more than 5% or in a 220V network - no more than 11V.
Therefore, the longer the cable, the larger its cross-section should be. You can determine it using tables or using an online calculator. In contrast to the choice of cross-section based on permissible heating, voltage losses do not depend on laying conditions and insulation material.
In a 220V network, voltage is supplied through two wires: phase and neutral, so the calculation is made using double the length of the cable. In the cable from the previous example it will be U=I*R=30A*2*0.074Ohm=4.44V. This is not much, but with a length of 25 meters it turns out to be 11.1V - the maximum permissible value, you will have to increase the cross-section.
Maximum permissible cable length of a given section
Electrical resistance of other metals
Current resistance: formula
In addition to copper and aluminum, other metals and alloys are used in electrical engineering:
- Iron. Steel has a higher resistivity, but is stronger than copper and aluminum. Steel strands are woven into cables designed to be laid through the air. The resistance of iron is too high to transmit electricity, so the core cross-sections are not taken into account when calculating the cross-section. In addition, it is more refractory, and leads are made from it for connecting heaters in high-power electric furnaces;
- Nichrome (an alloy of nickel and chromium) and fechral (iron, chromium and aluminum). They have low conductivity and refractoriness. Wirewound resistors and heaters are made from these alloys;
- Tungsten. Its electrical resistance is high, but it is a refractory metal (3422 °C). It is used to make filaments in electric lamps and electrodes for argon-arc welding;
- Constantan and manganin (copper, nickel and manganese). The resistivity of these conductors does not change with changes in temperature. Used in high-precision devices for the manufacture of resistors;
- Precious metals – gold and silver. They have the highest specific conductivity, but due to their high price, their use is limited.
Inductive reactance
Formulas for calculating the conductivity of wires are valid only in a direct current network or in straight conductors at low frequencies.
Inductive reactance appears in coils and in high-frequency networks, many times higher than usual. In addition, high frequency current only travels along the surface of the wire.
Therefore, it is sometimes coated with a thin layer of silver or Litz wire is used.
Reference. Litz wire is a stranded wire, each core in which is isolated from the rest. This is done to increase surface area and conductivity in high frequency networks.
Copper's resistivity, flexibility, relatively low price and mechanical strength make this metal, along with aluminum, the most common material for making wires.
Electrical resistance
Electrical resistance, one of the components of Ohm's law, is expressed in ohms (Ohms). It should be noted that electrical resistance and resistivity are not the same thing. Resistivity is a property of a material, while electrical resistance is a property of an object.
The electrical resistance of a resistor is determined by a combination of its shape and the resistivity of the material from which it is made.
For example, a wirewound resistor made from a long, thin wire has a higher resistance than a resistor made from a short, thick wire of the same metal.
At the same time, a wirewound resistor made of a high resistivity material has greater electrical resistance than a resistor made of a low resistivity material. And all this despite the fact that both resistors are made of wire of the same length and diameter.
To illustrate this, we can draw an analogy with a hydraulic system, where water is pumped through pipes.
- The longer and thinner the pipe, the greater the resistance to water.
- A pipe filled with sand will resist water more than a pipe without sand.
Electrical resistance of current.
Electrical resistance is a physical quantity that characterizes the ability of a conductor to influence the electric current flowing in the conductor.
- Quantity designation: R
- Unit: Ohm
As a result of experiments with conductors, it was determined that the relationship between current strength and voltage in an electrical circuit also depends on the size of the conductor used, and not just on the substance. The influence of conductor sizes will be discussed in more detail in a separate lesson.
What causes current resistance ? During the movement of free electrons, constant interaction occurs between the ions included in the structure of the crystal lattice and the electrons. As a result of this interaction, the movement of electrons slows down (in fact, due to the collision of electrons with atoms - nodes of the crystal lattice), due to which current resistance is created.
Another physical quantity is also associated with electrical resistance - current conductivity , the reciprocal of resistance.
Wire resistance
The amount of wire resistance depends on three parameters: the resistivity of the metal, the length and diameter of the wire itself. Formula for calculating wire resistance:
where: R - wire resistance (Ohm) ρ - metal resistivity (Ohm.m) L - wire length (m) A - cross-sectional area of the wire (m2)
As an example, consider a nichrome wirewound resistor with a resistivity of 1.10×10-6 Ohm.m. The wire has a length of 1500 mm and a diameter of 0.5 mm. Based on these three parameters, we calculate the resistance of the nichrome wire:
R=1.1*10 -6 *(1.5/0.000000196) = 8.4 Ohm
Nichrome and constantan are often used as resistance materials. Below in the table you can see the resistivity of some of the most commonly used metals.
Calculation using resistivity
Conductor resistance can be calculated without measuring voltage and current values. But for this you need to know additional information about the conductor.
Rice. 3. A conductor with cross-section S and length L through which current I flows.
Georg Ohm and other researchers experimentally determined that the resistance of a conductor is directly proportional to the length of the conductor L and inversely proportional to the cross-sectional area of the conductor S. This pattern can be described by the formula for calculating the resistance of the conductor:
$ R = ρ *{ L\over S} $ (2)
The coefficient ρ was called resistivity. This physical quantity reflects the characteristics of a particular substance, which depend on the density of the substance, crystal structure, atomic structure and other internal parameters. It is not necessary to calculate the resistivity of the conductor every time, since for most substances the resistivities are measured and summarized in reference tables, which can be found in paper reference books or in their online versions.
But if such a need arises, then from formula (2) you can obtain the following formula (3), and use it to calculate ρ:
$ ρ = R*{ S\over L } $ (3)
Silver has one of the lowest ρ values, equal to $0.016{Ohm*mm^2\over m}$. This explains the use of such a rather expensive metal for soldering especially important radio components (microcircuits, microprocessors, electronic boards), which should heat up as little as possible during operation.
Surface resistance
The surface resistance value is calculated in the same way as the wire resistance. In this case, the cross-sectional area can be represented as the product of w and t:
For some materials, such as thin films, the relationship between resistivity and film thickness is called sheet sheet resistance RS: where RS is measured in ohms. For this calculation, the film thickness must be constant.
Often, resistor manufacturers cut tracks into the film to increase resistance to increase the path for electrical current.
Properties of resistive materials
The resistivity of a metal depends on temperature. Their values are usually given for room temperature (20°C). The change in resistivity as a result of a change in temperature is characterized by a temperature coefficient.
For example, thermistors (thermistors) use this property to measure temperature. On the other hand, in precision electronics, this is a rather undesirable effect. Metal film resistors have excellent temperature stability properties. This is achieved not only due to the low resistivity of the material, but also due to the mechanical design of the resistor itself.
Many different materials and alloys are used in the manufacture of resistors. Nichrome (an alloy of nickel and chromium), due to its high resistivity and resistance to oxidation at high temperatures, is often used as a material for making wirewound resistors. Its disadvantage is that it cannot be soldered. Constantan, another popular material, is easy to solder and has a lower temperature coefficient.
Rheostats
A rheostat is a device that is used to regulate the current in a circuit.
The simplest rheostat is a wire with high resistivity, such as nickel or nichrome.
Types of rheostats:
A slider rheostat is another type of rheostat in which a steel wire is wound around a ceramic cylinder. The wire is covered with a thin layer of scale, which does not conduct electric current, so its turns are insulated from each other. Above the winding is a metal rod along which the slider moves.
It is pressed against the turns of the winding. From the friction of the slider on the turns, the scale layer is erased and the electric current in the circuit passes from the turns of wire to the slider, then into the rod. When the rheostat is connected to the circuit, you can move the slider, thus increasing or decreasing the resistance of the rheostat.
Liquid rheostat - represents a tank with electrolyte into which metal plates are immersed.
Wire rheostat - consists of a wire made of a material with high resistivity, stretched over a frame.
Do not exceed the current strength of the rheostat, because the rheostat winding may burn out.
We often use a rheostat in everyday life, for example, adjusting the volume of the TV and radio, increasing and decreasing the speed of driving a car.