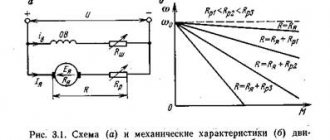
Magnet interaction
a north pole on two sides of each magnet.
and
the south pole
.
Two magnets are attracted to each other by opposite poles and repelled by like poles. Magnets can act on each other even through a vacuum! All this resembles the interaction of electric charges, but the interaction of magnets is not electrical
. This is evidenced by the following experimental facts.
• Magnetic force weakens as the magnet heats up. The strength of the interaction of point charges does not depend on their temperature.
• Magnetic force weakens when the magnet is shaken. Nothing like this happens with electrically charged bodies.
• Positive electrical charges can be separated from negative ones (for example, when electrifying bodies). But it is impossible to separate the poles of a magnet: if you cut a magnet into two parts, then poles also appear at the cut site, and the magnet splits into two magnets with opposite poles at the ends (oriented in exactly the same way as the poles of the original magnet).
Thus, magnets are always
bipolar, they exist only as
dipoles
.
Isolated magnetic poles (so-called magnetic monopoles
- analogues of electric charge) do not exist in nature (in any case, they have not yet been discovered experimentally). This is perhaps the most striking asymmetry between electricity and magnetism.
• Like electrically charged bodies, magnets act on electric charges. However, a magnet only acts on a moving
charge; if the charge is at rest relative to the magnet, then the effect of magnetic force on the charge is not observed. On the contrary, an electrified body acts on any charge, regardless of whether it is at rest or in motion.
According to modern concepts of the theory of short-range interaction, the interaction of magnets is carried out through a magnetic field
Namely, a magnet creates a magnetic field in the surrounding space, which acts on another magnet and causes a visible attraction or repulsion of these magnets.
An example of a magnet is a magnetic needle
compass. Using a magnetic needle, you can judge the presence of a magnetic field in a given region of space, as well as the direction of the field.
Our planet Earth is a giant magnet. Not far from the north geographic pole of the Earth is the south magnetic pole. Therefore, the north end of the compass needle, turning towards the south magnetic pole of the Earth, points to geographic north. This is where the name “north pole” of a magnet came from.
Magnetic field lines
The electric field, we recall, is studied using small test charges, by the effect on which one can judge the magnitude and direction of the field. The analogue of a test charge in the case of a magnetic field is a small magnetic needle.
For example, you can get some geometric insight into the magnetic field by placing very small compass needles at different points in space. Experience shows that the arrows will line up along certain lines - the so-called magnetic field lines
. Let us define this concept in the form of the following three points.
1. Magnetic field lines, or magnetic lines of force, are directional lines in space that have the following property: a small compass needle placed at each point on such a line is oriented tangent to this line
.
2. The direction of the magnetic field line is considered to be the direction of the northern ends of the compass needles located at points on this line
.
3. The denser the lines, the stronger the magnetic field in a given region of space.
.
Iron filings can successfully serve as compass needles: in a magnetic field, small filings become magnetized and behave exactly like magnetic needles.
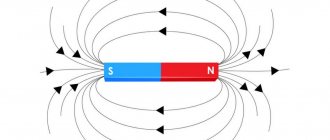
So, by pouring iron filings around a permanent magnet, we will see approximately the following picture of magnetic field lines (Fig. 1).
Rice. 1. Permanent magnet field
The north pole of a magnet is indicated by the color blue and the letter ; the south pole - in red and the letter . Please note that the field lines leave the north pole of the magnet and enter the south pole: after all, it is towards the south pole of the magnet that the north end of the compass needle will be directed.
Model of the magnetic field of a moving charge
To remember the direction of the magnetic field of a moving positive charge, we will imagine ourselves in its place.
Raise your right hand up, then point it to the right, then lower it down, then point it to the left and return the hand to its original position - up. Then we repeat this movement. Our hand makes circles clockwise. Now let's start moving forward, continuing to rotate our hand. The movement of our body is an analogue of the movement of a positive charge, and the rotation of our hand clockwise is an analogue of the magnetic field of a charge. Now imagine that around us there is a thin and strong elastic web, similar to the strings of space that we drew when creating a model of the electric field.
When we move through this three-dimensional “web”, due to the rotation of the hand, it, deformed, moves clockwise, forming a kind of spiral, as if wound in a coil around a charge. Behind us, the “web” is restoring its correct structure. This is roughly how you can imagine the magnetic field of a positive charge moving straight.
Now try to move not straight forward, but in a circle, for example, turning left while walking, while rotating your hand clockwise. Imagine that you are moving through something that resembles jelly. Due to the rotation of your hand, inside the circle you are moving in, the “jelly” will move upward, forming a hump above the center of the circle. And under the center of the circle, a depression is formed due to the fact that part of the jelly has shifted upward. This is how you can imagine the formation of the north (hump on top) and south (hollow on the bottom) poles when a charge moves around the ring or rotates.
If you turn right while walking, a “hump” (north pole) will form at the bottom.
Similarly, one can form an idea of the magnetic field of a moving negative charge. You just need to rotate your hand in the opposite direction - counterclockwise. Accordingly, the magnetic field will be directed in the opposite direction. Just watch each time in which direction your hand pushes the “jelly”.
This model clearly demonstrates why the north pole of one magnet is attracted to the south pole of another magnet: the “hump” of one magnet is pulled into the “hollow” of the second magnet.
And this model also shows why there are no separate north and south poles of magnets, no matter how we cut them - the magnetic field is a vortex (closed) “deformation of space” around the trajectory of a moving charge.
Oersted's experience
Despite the fact that electrical and magnetic phenomena have been known to people since antiquity, no relationship between them was observed for a long time. For several centuries, research into electricity and magnetism proceeded in parallel and independently of each other.
The remarkable fact that electrical and magnetic phenomena are actually related to each other was first discovered in 1820 in Oersted's famous experiment.
The diagram of Oersted's experiment is shown in Fig. 2 (image from the site rt.mipt.ru). Above the magnetic needle (and are the north and south poles of the needle) there is a metal conductor connected to a current source. If you close the circuit, the arrow turns perpendicular to the conductor! This simple experiment directly indicated the relationship between electricity and magnetism. The experiments that followed Oersted's experiment firmly established the following pattern: the magnetic field is generated by electric currents and acts on the currents
.
Rice. 2. Oersted's experiment
The pattern of magnetic field lines generated by a current-carrying conductor depends on the shape of the conductor.
Magnetic field of a straight wire carrying current
The magnetic field lines of a straight wire carrying current are concentric circles. The centers of these circles lie on the wire, and their planes are perpendicular to the wire (Fig. 3).
Rice. 3. Field of a straight wire with current
There are two alternative rules for determining the direction of forward magnetic field lines.
Clockwise rule
.
The field lines go counterclockwise if you look so that the current flows towards us
.
Screw rule
(or
the gimlet rule
, or
the corkscrew rule
- whichever is closer ;-)).
The field lines go where you need to rotate the screw (with a regular right-hand thread) so that it moves along the thread in the direction of the current
.
Use the rule that suits you best. It is better to get used to the clockwise rule - you will later see for yourself that it is more universal and easier to use (and then remember it with gratitude in your first year, when you study analytical geometry).
In Fig. 3 something new has appeared: this is a vector, which is called the magnetic field induction
, or
magnetic induction
.
The magnetic induction vector is an analogue of the electric field strength vector: it serves as a force characteristic
of the magnetic field, determining the force with which the magnetic field acts on moving charges.
We will talk about forces in a magnetic field later, but for now we will only note that the magnitude and direction of the magnetic field is determined by the magnetic induction vector. At each point in space, the vector is directed in the same direction as the northern end of the compass needle placed at a given point, namely, tangent to the field line in the direction of this line. Magnetic induction is measured in tesla
(Tl).
As in the case of an electric field, the principle of superposition
.
It lies in the fact that the induction of magnetic fields created at a given point by various currents add up vectorially and give the resulting vector of magnetic induction:
.
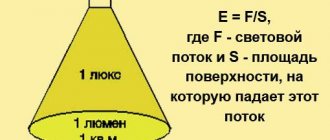
How to measure magnetic field?
Due to the fact that the magnetic field is a vector quantity, to describe it you need to know two characteristics: strength and direction. Direction is easier to measure. For example, use a compass whose arrow coincides with the field lines at a given point. Compasses began to be used in the 10th-11th centuries.
Measuring strength is much more difficult. Instruments capable of measuring magnetic force (magnetometers) began to be used only in the 19th century. The basic principle of operation of such a device is the use of the force experienced by an electron moving in a magnetic field. In the SI system, the magnetic field is expressed in teslas (T)(T)(T).
Definition of Tesla
This is a unit of measurement of magnetic field induction, equal to the induction of a uniform magnetic field, in which a force of 1 newton acts on 1 meter of a straight conductor perpendicular to the magnetic induction vector with a current of 1 ampere.
In other words, Tesla shows how much force is applied to a moving charge due to the action of the field. An alternative unit of measurement for magnetic field is gauss (G s) (G) (G). 1 T = 1 0 4 Gs 1~T = 10^4 ~Gs 1 T=104 Gs. Gauss is more commonly used since 1 Tesla is an extremely large field.
In the equations, the magnitude of the magnetic field is represented by the symbol BBB, but you will also notice that another quantity is used, HH H. Both quantities have the same units, but HHH takes into account the effect of the density of magnetic fields in magnetic materials. For most problems in which calculations occur in a vacuum, the value BB B is sufficient.
Magnetic field of a coil with current
Consider a circular coil through which a direct current circulates. We do not show the source that creates the current in the figure.
The picture of the field lines of our orbit will look approximately as follows (Fig. 4).
Rice. 4. Field of a coil with current
It will be important for us to be able to determine into which half-space (relative to the plane of the coil) the magnetic field is directed. Again we have two alternative rules.
Clockwise rule
.
The field lines go there, looking from where the current appears to circulate counterclockwise
.
Screw rule
.
The field lines go where the screw (with a normal right-hand thread) will move if rotated in the direction of the current
.
As you can see, the roles of the current and the field are reversed, compared to the formulation of these rules for the case of direct current.
Magnetic field of a current coil
Coil
It will work if you wind the wire tightly, turn to turn, into a sufficiently long spiral (Fig. 5 - image from en.wikipedia.org).
The coil may have several tens, hundreds or even thousands of turns. The coil is also called a solenoid
.
Rice. 5. Coil (solenoid)
The magnetic field of one turn, as we know, does not look very simple. Fields? individual turns of the coil are superimposed on each other, and it would seem that the result should be a very confusing picture. However, this is not so: the field of a long coil has an unexpectedly simple structure (Fig. 6).
Rice. 6. current coil field
In this figure, the current in the coil flows counterclockwise when viewed from the left (this will happen if in Fig. 5 the right end of the coil is connected to the “plus” of the current source, and the left end to the “minus”). We see that the magnetic field of the coil has two characteristic properties.
1. Inside the coil, away from its edges, the magnetic field is uniform
: at each point the magnetic induction vector is the same in magnitude and direction. Field lines are parallel straight lines; they bend only near the edges of the coil when they come out.
2. Outside the coil the field is close to zero. The more turns in the coil, the weaker the field outside it.
Note that an infinitely long coil does not release the field outward at all: there is no magnetic field outside the coil. Inside such a coil, the field is uniform everywhere.
Doesn't remind you of anything? A coil is the “magnetic” analogue of a capacitor. You remember that a capacitor creates a uniform electric field inside itself, the lines of which bend only near the edges of the plates, and outside the capacitor the field is close to zero; a capacitor with infinite plates does not release the field to the outside at all, and the field is uniform everywhere inside it.
And now - the main observation. Please compare the picture of the magnetic field lines outside the coil (Fig. 6) with the magnet field lines in Fig. 1. It's the same thing, isn't it? And now we come to a question that has probably arisen in your mind for a long time: if a magnetic field is generated by currents and acts on currents, then what is the reason for the appearance of a magnetic field near a permanent magnet? After all, this magnet does not seem to be a conductor with current!
Magnetic field and its properties
A magnetic field is matter that occurs around sources of electric current, as well as around permanent magnets. In space, the magnetic field is displayed as a combination of forces that can influence magnetized bodies. This action is explained by the presence of driving discharges at the molecular level.
A magnetic field is formed only around electric charges that are in motion. That is why the magnetic and electric fields are integral and together form the electromagnetic field. The components of the magnetic field are interconnected and influence each other, changing their properties.
Properties of a magnetic field: 1. A magnetic field arises under the influence of driving charges of electric current. 2. At any point, a magnetic field is characterized by a vector of a physical quantity called magnetic induction, which is a force characteristic of a magnetic field. 3. A magnetic field can only affect magnets, current-carrying conductors and moving charges. 4. The magnetic field can be of a constant or variable type 5. The magnetic field is measured only by special instruments and cannot be perceived by human senses. 6. The magnetic field is electrodynamic, since it is generated only by the movement of charged particles and affects only charges that are in motion. 7. Charged particles move along a perpendicular trajectory.
The size of the magnetic field depends on the rate of change of the magnetic field. According to this feature, there are two types of magnetic field: dynamic magnetic field and gravitational magnetic field. The gravitational magnetic field arises only near elementary particles and is formed depending on the structural features of these particles. A magnetic moment occurs when a magnetic field acts on a conductive frame. In other words, the magnetic moment is a vector that is located on the line that runs perpendicular to the frame.
The magnetic field can be represented graphically using magnetic field lines. These lines are drawn in such a direction that the direction of the field forces coincides with the direction of the field line itself. Magnetic lines of force are continuous and closed at the same time.
The direction of the magnetic field is determined using a magnetic needle. The lines of force also determine the polarity of the magnet, the end with the output of the force lines is the north pole, and the end with the input of these lines is the south pole.
It is very convenient to visually evaluate the magnetic field using ordinary iron filings and a piece of paper. If we put a sheet of paper on a permanent magnet and sprinkle sawdust on top, then the iron particles will line up according to the magnetic field lines.
The direction of the field lines for a conductor is conveniently determined by the famous gimlet rule or the right-hand rule. If we wrap our hand around the conductor so that the thumb points in the direction of the current (from plus to minus), then the 4 remaining fingers will show us the direction of the magnetic field lines. And the direction of the Lorentz force is the force with which the magnetic field acts on a charged particle or conductor with current, according to the left-hand rule. If we place our left hand in a magnetic field so that 4 fingers look in the direction of the current in the conductor, and the lines of force enter the palm, then the thumb will indicate the direction of the Lorentz force, the force acting on the conductor placed in the magnetic field.
That's all. Be sure to ask any questions you have in the comments.
Note: learning English? — the rating of English language schools (https://www.schoolrate.ru/) will be useful to you when choosing.
If the material was useful, you can
send a donation or share this material on social networks: