Instantaneous value
2015-04-23 7116 The instantaneous value of the quantity a is written in the form:
where Am is the amplitude (maximum value) of the quantity;
ω – angular frequency, rad/s;
t – current time value, s;
ψ – initial phase.
We write the instantaneous values of current i, voltage u or EMF in the form:
The sine argument (ωt +ψ) is called the phase
.
The angle ψ is equal to the phase at the initial moment of time t = 0 and is therefore called the initial phase
.
Angular frequency ω is related to period T and frequency f =1/T by the formulas:
ω = 2π/Т or ω = 2πf.
Frequency f, equal to the number of oscillations per second, is measured in hertz (Hz). At f=50 Hz we have ω =314 rad/s.
Effective and average values of sinusoidal currents and voltages
The effective value of the sinusoidal current
is equal to the value of the direct current, which in one period generates the same amount of heat in the same resistor as the sinusoidal current.
Similarly, there are concepts of effective values of sinusoidal voltages and EMF:
And .
The effective value of a sinusoidal current is often called the root mean square or effective value.
The effective values of currents and voltages are shown by most electrical measuring instruments (ammeters, voltmeters).
The current values indicate the rated currents and voltages in the passports of various electrical appliances and devices.
Below the average
sinusoidal current understand its average value over half a period:
and
Elements of electrical circuits of sinusoidal current
Basic elements of electrical circuits of sinusoidal current:
- sources of electrical energy (EMF sources and current sources);
- resistive elements (resistors, rheostats, heating elements, etc.);
-capacitive elements (capacitors);
- inductive elements (inductors).
Resistive element
According to Ohm's law, the voltage on the resistive element is: u=i⋅R=R⋅Im sinωt=Um sinωt, where Um =R⋅Im and current i=Im sinωt.
1. Current and voltage in a resistive element are in phase (change in phase).
2. Ohm’s law holds true for both amplitude values of current and voltage:
Um =R⋅Im, and for effective values of current and voltage: U=R⋅I.
Let us express the instantaneous power p in terms of the instantaneous values of current i and voltage u:
Source
Ampere is a unit of current in the International System of Units.
The SI unit of current is the ampere. It is denoted by the letter A. One ampere is the strength of such a direct electric current that a charge equal to one coulomb passes through the cross-section of the conductor in one second:
The ampere (unit of current) is one of the seven SI base units. Back in 1948, the definition of the unit of current was the interaction of a pair of parallel conductors through which currents flow. We remember that when currents flowing through two parallel conductors have the same directions, they attract; if the currents are in opposite directions, then the conductors repel, and the Ampere force arises. One ampere is defined using the concept of ampere power. They say that an ampere is the strength of a current passing in two straight, infinitely thin and long conductors located in a vacuum, generating an Ampere force (the force of interaction of conductors) equal to $2\cdot ^H$ for each meter of conductor.
The ampere received its name in honor of the French physicist A.M. Ampere.
One ampere is quite a lot of current. It is believed that for a person the current strength becomes dangerous from 0.001 A, and the current strength from 0.1 A can cause significant harm to health. In practical calculations, multiple and submultiple units of current are used, using standard SI prefixes. For example, microampere $1μA=^A;;kiloampere\ 1kA=1000\ A.$
War of Currents
Active use of direct current began at the end of the 19th century. Then Edison perfected the light bulb (1890) and founded the first power plants in New York that produced 110 volt direct current.
The use of direct current was associated with significant losses when transmitting it over long distances. Alternating current could not be used due to the lack of adequate meters and motors that operated on alternating current. The process of converting direct current into alternating current was also difficult. At the same time, alternating current could be transmitted over long distances without loss.
At that time, Nikola Tesla came to America from Serbia and got a job at Edison’s company. Tesla invented the alternating current electric motor, realized all the benefits and suggested its use to Edison.
Tesla and Edison
Edison did not listen to Tesla and also did not pay him his salary. Thus began the famous confrontation between inventors - the war of currents.
It lasted more than a hundred years and ended in 2007. Then New York completely switched to alternating current electricity.
Units of current in other systems of units
In the system of units, which is an extension of the SGS and is called SGSM (absolute electromagnetic system centimeter, gram, second), bio (abamper) is a unit of measurement of current strength.
One bio (abamper) is a current of such strength that it creates an Ampere force equal to 2 dynes for every centimeter of the length of the conductor, which occurs between two thin, long parallel conductors located at a distance of 1 cm, through which currents flow.
The current strength (bio) in the SGSM system was named after the French scientist J.B. Biot. Sometimes in the SGSM system the current measurement unit is not named and is simply called the SGSM current measurement unit (this unit is equivalent to the bio unit and abamper (abA)).
In another extension of the CGS system, the SGSE system (absolute electrostatic system centimeter, gram, second), the statampere is a unit of current. The statampere (statA) is defined as the current strength at which, in a time equal to one second, a charge of 1 statcoulomb passes through the cross-section of the conductor.
\[1\ A=2997924536.843\ statA.\]
General definitions
The physical process in which charged particles move in an orderly (directional) manner is called electric current. It is usually divided into variable and constant. For the first, the direction and magnitude remain unchanged, but for the second, these characteristics change according to a certain pattern.
The above definitions are greatly simplified, although they explain the difference between direct and alternating current. To better understand what this difference is, it is necessary to provide a graphical representation of each of them, as well as explain how the alternating electromotive force is generated in the source. To do this, let us turn to electrical engineering, or rather its theoretical foundations.
Examples of problems with solutions
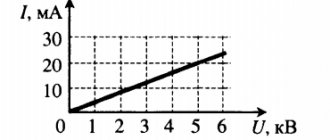
Exercise. What was the average current ($\left\langle I\right\rangle $) if a capacitor with a capacity of $C=100 µF$ was charged to a voltage of $U=500$V in a time of $\Delta t=$0.5 s? In what units will the resulting current be measured?\textit
Solution. We define the average current strength as:
\[\left\langle I\right\rangle =\frac\left(1.1\right).\]
We find the charge received by the capacitor as:
\[\Delta q=C\cdot U\left(1.2\right).\]
A Brief History of Electricity
Who invented electricity? And no one! People gradually understood what it was and how to use it.
It all started in the 7th century BC, on one sunny (or maybe rainy, who knows) day. Then the Greek philosopher Thales noticed that if you rub amber on wool, it will attract light objects.
Then there were Alexander the Great, wars, Christianity, the fall of the Roman Empire, wars, the fall of Byzantium, wars, the Middle Ages, the Crusades, epidemics, the Inquisition and more wars. As you understand, people had no time for any electricity or ebonite sticks rubbed with wool.
In what year was the word “electricity” invented? In 1600, the English naturalist William Gilbert decided to write the work “On the Magnet, Magnetic Bodies and the Great Magnet - the Earth.” “electricity” appeared .
One hundred and fifty years later, in 1747, Benjamin Franklin, whom we all love very much, created the first theory of electricity. He viewed this phenomenon as a fluid or immaterial liquid.
It was Franklin who introduced the concept of positive and negative charges (before that, glass and resin electricity were separated), invented the lightning rod and proved that lightning is electrical in nature.
Everyone loves Benjamin, because his portrait is on every hundred dollar bill. In addition to his work in the exact sciences, he was a prominent political figure. But contrary to popular belief, Franklin was not the President of the United States.
Next will be a list of discoveries important for the history of electricity.
1785 - Coulomb finds out with what force opposite charges attract and like charges repel.
1791 - Luigi Galvani accidentally noticed that the legs of a dead frog contracted under the influence of electricity.
The operating principle of the battery is based on galvanic cells. But who created the first galvanic cell? Based on Galvani's discovery, another Italian physicist Alessandro Volta created the Volta column in 1800, the prototype of the modern battery.
At excavations near Baghdad, they found a battery more than two thousand years old. What ancient iPhone was recharged with its help remains a mystery. But we know for sure that the battery has already run out. This case seems to say: maybe people knew about electricity much earlier, but then something went wrong.
Alternating electric current
In a mechanical system, forced vibrations occur when an external periodic force acts on it. Similarly, forced electromagnetic oscillations in an electrical circuit occur under the influence of an external periodically varying EMF or an externally varying voltage.
Forced electromagnetic oscillations in an electrical circuit represent alternating electric current
.
- Alternating electric current
is a current whose strength and direction periodically change.
In the future, we will study forced electrical oscillations that occur in circuits under the influence of voltage that varies harmoniously with frequency ω
according to the sinusoidal or cosine law:
u = U_m \cdot \sin \omega t\) or \(
u = U_m \cdot \cos \omega t\) ,
where u
– instantaneous voltage value,
U
m – voltage amplitude, ω – cyclic oscillation frequency. If the voltage changes with a frequency ω, then the current in the circuit will change with the same frequency, but the current fluctuations do not necessarily have to be in phase with the voltage fluctuations. Therefore, in the general case
i = I_m \cdot \sin (\omega t + \varphi_c)\) ,
where φc is the phase difference (shift) between current and voltage fluctuations.
Based on this, we can give the following definition:
- Alternating current
is an electric current that changes over time according to a harmonic law.
Alternating current ensures the operation of electric motors in machines in plants and factories, powers lighting fixtures in our apartments and outdoors, refrigerators and vacuum cleaners, heating appliances, etc. The frequency of voltage fluctuations in the network is 50 Hz. The alternating current has the same oscillation frequency. This means that within 1 s the current will change its direction 50 times. A frequency of 50 Hz is accepted for industrial current in many countries around the world. In the USA, the frequency of industrial current is 60 Hz.
D.C
Direct Current or DC in English means an electric current that does not change direction over any period of time and always moves from plus to minus. In the diagram it is indicated as plus (+) and minus (-); on the body of a device operating on direct current, a designation is applied in the form of one (-) or (=) stripe. An important feature of direct electric current is the possibility of its accumulation, i.e. accumulation in batteries or obtaining it due to a chemical reaction in batteries. Many modern portable electrical devices operate using accumulated direct current electrical charge, which is located in the batteries of these same devices.
Alternator
The bulk of the world's electricity is currently generated by alternating current generators, which create harmonic oscillations.
- An alternating current generator
is an electrical device designed to convert mechanical energy into alternating current energy.
The induction emf of the generator changes according to a sinusoidal law
where \(_ =B\cdot S\cdot \omega\) is the amplitude (maximum) value of the EMF. When connected to the terminals of the load frame with resistance R
, alternating current will pass through it. According to Ohm's law for a section of a circuit, the current in the load
where \(I_ = \dfrac\) is the amplitude value of the current.
The main parts of the generator are (Fig. 1):
- inductor
- an electromagnet or permanent magnet that creates a magnetic field; - armature
- a winding in which an alternating EMF is induced; - a commutator with brushes
is a device by which current is removed from or supplied to rotating parts.
The stationary part of the generator is called the stator
, and the movable one is
the rotor
. Depending on the design of the generator, its armature can be either a rotor or a stator. When receiving high-power alternating currents, the armature is usually made motionless in order to simplify the current transmission circuit to the industrial network.
In modern hydroelectric power plants, water rotates the shaft of an electric generator at a frequency of 1-2 revolutions per second. Thus, if the generator armature had only one frame (winding), then an alternating current with a frequency of 1-2 Hz would be obtained. Therefore, to obtain alternating current with an industrial frequency of 50 Hz, the armature must contain several windings that allow increasing the frequency of the generated current. For steam turbines, the rotor of which rotates very quickly, an armature with one winding is used. In this case, the rotor rotation frequency coincides with the alternating current frequency, i.e. the rotor should make 50 rps.
Powerful generators produce a voltage of 15-20 kV and have an efficiency of 97-98%.
From the history
. Initially, Faraday detected only a barely noticeable current in the coil when a magnet moved near it. "What's the use of this?" - they asked him. Faraday replied: “What use can a newborn baby have?” A little more than half a century has passed and, as the American physicist R. Feynman said, “the useless newborn turned into a miracle hero and changed the face of the Earth in a way that his proud father could not even imagine.”
*Operating principle
The operating principle of an alternating current generator is based on the phenomenon of electromagnetic induction.
Let a conducting frame of area S
rotates with angular velocity ω around an axis located in its plane perpendicular to a uniform magnetic field with induction \(\vec
\) (see Fig. 1).
Principle of operation
Mechanical energy is converted by the generator shown in the figure into electrical energy as follows:
Due to such a phenomenon as electromagnetic induction, when the frame “4” rotates, placed in the magnetic field “3” (arising between the different poles of the magnet “2”), an emf “5” is formed in it. Voltage is supplied to the network through current collectors “7” from ring contacts “6”, to which frame “4” is connected.
Video: direct and alternating current - differences
As for the magnitude of the EMF, it depends on the speed of intersection of the power lines “3” by the frame “4”. Due to the characteristics of the electromagnetic field, the minimum crossing speed, and therefore the lowest value of the electromotive force, will be at the moment when the frame is in a vertical position, respectively, the maximum - in a horizontal position.
RMS values of current and voltage
Let the current source create an alternating harmonic voltage
According to Ohm's law, the current in a section of a circuit containing only a resistor with a resistance R
, connected to this source, also changes with time according to a sinusoidal law:
where \(I_m = \dfrac>.\) As we see, the current strength in such a circuit also changes over time according to a sinusoidal law. Quantities Um
,
Im
are called
the amplitude values of voltage and current
.
The time-dependent values of voltage u
and current
i
are called
instantaneous
.
In addition to these quantities, another characteristic of alternating current is used: effective (effective) values of current and voltage
.
- The effective (effective) value of an
alternating current is the strength of a direct current that, passing through a circuit, releases the same amount of heat per unit time as a given alternating current.
Denoted by the letter I
.
- The effective (effective) value of an
alternating current voltage is the voltage of a direct current that, passing through a circuit, releases the same amount of heat per unit time as a given alternating current.
Denoted by the letter U
.
Active ( I, U
) and amplitude (
Im, Um
) values are related to each other by the following relationships:
Thus, the expressions for calculating the power consumed in direct current circuits remain valid for alternating current if we use the effective values of current and voltage in them:
It should be noted that Ohm's law for an alternating current circuit containing only a resistor with a resistance R
, is carried out both for amplitude and effective, and for instantaneous values of voltage and current, due to the fact that their oscillations coincide in phase.
*Derivation of the formula
Knowing the instantaneous values of u
and
i
, the instantaneous power can be calculated
which, unlike direct current circuits, changes over time. Taking into account equations (1) and (2), we rewrite the expression for instantaneous power on the resistor in the form
The first term does not depend on time. Second term P
2 - the cosine function of double the angle and its average value over the period of oscillation is zero (Fig. 2, find the sum of the area of the selected figures, taking into account the signs).
Therefore, the average value of the alternating electric current power over the period will be equal to
Then, taking into account Ohm’s law \(\left(I_ =\dfrac> \right)\) we obtain:
To determine the effective values, it is necessary to compare the power (amount of heat per unit time) of alternating and direct current. Let's write down the equations to calculate DC power
and compare with equations (4>:
Alternating and direct current: what is the difference, history of development, application
Entrust your work to a PhD candidate!
Children are taught that they should not stick their fingers into electrical sockets! And why? Because it will be bad. There are often problems with a more detailed explanation: there is some kind of voltage, current, something is flowing somewhere. So that in the future you can explain to your children what’s what, we will now explain to you. This article is about alternating and direct currents, their differences, applications and the history of electricity in general. Science needs to be made interesting, and we modestly try to do this to the best of our ability.
For example: what current is in our sockets? Variable, of course! Voltage 220 Volts and frequency 50 Hertz. And the network through which the current is transmitted is three-phase. By the way, if at the words “phase” and “zero” you fall into a stupor, read what it is, and the day will be lived doubly not in vain! But let's not get ahead of ourselves. First things first.
Daily newsletter with useful information for students of all directions - on our telegram channel.