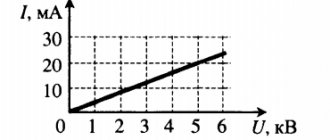
Traction formula
Message from the administrator:
In the application you can learn words, train listening and pronunciation.
Give it a try. Two lessons for free using my link! Click HERE
Traction force
can be determined in terms of net power and vehicle speed (v):
For a car going up a hill that has a slope
, the mass of the car m traction force (FT) will enter into the equation:
where a is the acceleration with which the car is moving.
Air flows during wing movement
Let us find out the origin of the lift force acting on the wing of the aircraft. We will assume that the wing is an asymmetrical body, which is blown by a horizontal air flow (Fig. 1).
Rice. 1
Let us position the wing relative to the flow so that the plane drawn along the wing through the most distant points of its profile (points aaa and bbb) forms an angle α α α with the direction of the flow, which is called the angle of attack. The quantity ab ab ab is called the chord of the wing. Since the velocities of air particles in the boundary layer increase as they move away from the surface of the wing, the air movement in this layer will be vortex. The vortices will rotate clockwise above the wing, and counterclockwise under the wing. Let's assume that some mass of air breaks away under the wing of an airplane and is carried away by the flow in the form of vortices. Their angular momentum is non-zero. In the wing-air system, internal interaction forces, that is, viscous friction forces and pressure forces, cannot change the total angular momentum. If it is equal to zero before the formation of vortices, then, according to the law of conservation of angular momentum, after the formation of vortices the angular momentum should not change. It follows from this that simultaneously with the formation of vortices, air circulation around the wing should occur in the direction opposite to the direction of rotation of the vortices. The angular momentum of air circulation is equal in magnitude to the angular momentum of the vortices, but opposite in direction. In this case, the total angular momentum of the entire system is zero.
In Fig. Figure 2 shows the profile of a wing located in the air flow. The flow lines of this flow are shown as solid lines, and the circulation flows are shown as dashed lines.
Rice. 2
So, as a result of the action of viscous forces when air flows asymmetrically around the wing, air circulation occurs around it. It is called an attached vortex. This circulation flow is added to the air flow towards the wing, causing the air speed above the wing to be greater than below the wing. In a circulation flow, gas particles are not in rotational motion, but move conventionally forward along closed trajectories.
Traction formula
If a body has acceleration when moving, then, in addition to all others, it is necessarily acted upon by a certain force, which is the traction force at the moment in time under consideration. In fact, if a body moves in a straight line and at a constant speed, then the traction force also acts, since the body must overcome resistance forces. Typically, the traction force is found by considering the forces acting on the body, finding the resultant and applying Newton's second law. There is no strictly defined formula for traction force.
It should not be assumed that the traction force of, for example, a vehicle acts from the engine, since internal forces cannot change the speed of the system as a whole, which would be in conflict with the law of conservation of momentum. However, it should be noted that in order to obtain the required direction from the static friction force, the motor rotates the wheels, the wheels “cling to the road” and a traction force is generated. Theoretically, it would be possible not to use the concept of “thrust force”, but to talk about the static friction force or the air reaction force. But it is more convenient to divide the external forces that act on transport into two parts, with some forces called traction forces
, and others - by resistance forces. This is done so that the equations of motion do not lose their universal form and the useful mechanical power (P) has a simple expression:
Concept of power
It arose in the works of ancient Greek scientists. It was rather of a philosophical nature and was quite confusing and ambiguous, which did not prevent ancient practicing engineers from making very accurate calculations, since the understanding of force as the cause of movement was unconditional for them.
Later, such titans as Roger Bacon and William Ockham (English philosophers and natural scientists) dealt with the problem. Again, without a strict physical approach, but with a deeper understanding of the topic (the theory of “long-range action”).
Backman, Descartes, Galileo argued against archaic theories. Classical mechanics was making its way.
Johannes Kepler also at first gave esoteric properties to the force. But observations of the patterns of movement of celestial bodies killed the bad theologian and gave birth to the scientist. The idea of the general force of gravity appeared logically. Before Newton, contrary to popular belief.
Newton summarized and combined previously accumulated knowledge. Established a formula for the relationship between the force acting on a body and its movement (II Law).
Where:
- F is the vector (there is also a direction of application) of the force. In the SI system adopted by the Russian Federation (ISQ) it is measured in Newtons (N, N in international writing);
- m – mass of a material point (kg);
- a is the vector of the resulting acceleration (m/s2).
However, no definition of force was given. This is understandable: the phenomenon does not exist by itself. The term appeared only for the convenience of calculations and implies a measure of the influence of a foreign body or field on the observed object.
It is possible that gravity is the action of a field. The law of universal gravitation was also introduced by Newton.
Where:
· G – gravitational constant;
· m1, m2 – masses of material points (kg);
· R – distance between objects (m).
The Earth's gravity is calculated using the traditional formula of Newton's II law. Only "a" changes to the gravitational acceleration "g".
For approximate calculations, g is taken equal to 9.81 m/s2, which corresponds to an average latitude of 45.5°. For accurate ones, use the corresponding tables.
Examples of problem solving
Exercise. A car with a mass of 1 ton, when moving on a horizontal surface, is subject to a friction force equal to
=0.1 from gravity. What will be the traction force if the car moves with an acceleration of 2 m/s?
Solution. Let's make a drawing.
As a basis for solving the problem, we use Newton’s second law:
Let's project equation (1.1) on the X and Y axes:
Substituting the right side of expression (1.4) instead of the friction force in (1.2), we obtain:
Let's convert the mass to the SI system m=1t=10 3 kg and carry out the calculations:
Answer. FT=2.98 kN
Psst.. Do you want to make money on marketplaces?
Find out what product is in demand, put it on the marketplace and start earning money! Follow my link and get free access to the analytics system ! Use promotional code VASILIK50 for a 50% discount on any tariff!
You can see how a particular seller earns, how many times and for what amount each specific product was sold. Evaluate niches in general and much more. The system includes training and webinars.
Give it a try. My link will provide free access for several days. Click HERE
Source
Law of Gravity
Remark 1
Gravity is a special case of the manifestation of the gravitational field.
The force of gravity is a force that acts on bodies with mass located near the planets and gives them the acceleration of free fall. The formula for calculating the acceleration of gravity is as follows:
\(g=G {M \over r^2 } ,\)
where \(G\) is the gravitational constant, G=6.7∙10-11 N∙m2/kg2;
\(M\) – mass of a planet or other cosmic body;
\(r\) – distance between the object and the planet.
Gravity is calculated using the following formula:
\(\overrightarrow{F_g} = m\overrightarrow{g}.\)
Gravity is a vector quantity, that is, it has a certain direction of action, namely towards the center of the planet or other celestial body. Since this force acts constantly near the planet, the object falls freely with constant acceleration. In this case, the trajectory of movement under the influence of gravity depends on several parameters:
- direction of the velocity vector;
- modular value of the initial speed.
We encounter the effects of gravity at every step in everyday life. This force is often confused with body weight, since the formula for calculating them is the same:
\(P=mg,\)
but the physical meaning of these forces is different. The force of weight arises under the influence of gravity, but its essence is that it characterizes the force of an object on a support, in contrast to the force of gravity acting on an object.
Did not you find what you were looking for?
Just write and we will help
If we consider the law of universal attraction formulated by Isaac Newton, it states that all bodies having a certain mass are attracted to each other under the influence of gravity:
\(F=G {m_1 m_2 \over r^2}.\)
Definition 1
Weight is the force exerted by a body on the surface of the planet, arising under the influence of gravity.
If an object moves at a uniform speed along a horizontal plane or is at rest, a support reaction force arises, which will be equal in value to the weight of the body:
\(P=τg.\)
In this case, it will also be equal to the force of gravity.
If an object moves uniformly accelerated along the vertical, its weight will not be equal in value to the force of gravity. Its value will depend on the direction of the acceleration vector. If it is directed opposite to the force of gravity, the object is in a state of overload. If the object moves downwards and at the same time \(a=g\), the weight is equal to zero, and the object is in weightlessness.
The gravitational field strength is determined by the formula:
\(g= {F \over m},\)
where \(F\) is the force of gravity acting on a material point with mass m.
The potential energy of gravitational interaction of two material points with masses \(m_1\) and \(m_2, \) located at a distance \(r\) is calculated as follows:
\(E_p=Gm_1 m_2 r.\)
Varieties of speed
The magnitude of the velocity force depends on time. According to the nature of the body's behavior, the speed of uniform and uneven movement is distinguished. Uniform motion is characterized by a constant speed, that is, one that does not change its value over time. Uneven movement is characterized by an average speed, that is, one that represents the average value of all instantaneous speeds of movement of the body.
Is it difficult to figure it out on your own?
Try asking your teachers for help
Solving problems Tests Essays
The average speed is calculated as follows:
\(\overrightarrow{U_{avg}} = {\overrightarrow{δs} \over δt}.\)
Instantaneous speed is a quantity that shows the speed of movement of a body at a certain moment in time; it is defined as the limit to which the average speed tends with an unlimited reduction in the time interval. Calculated using the following formula:
\(\overrightarrow{U} =\lim\limits_{δt \to 0} {\overrightarrow{δs} \over δt}. \)
Instantaneous speed is the ratio of a small distance \(\overrightarrow{δs}\) to a small moment of time \(δt,\) during which this distance was covered.
Normal reaction force calculator
The normal force calculator will help you find the force a surface exerts to prevent an object from falling through it. In the following text, we will provide you with some normal force formulas and the answer to a simple question: what is normal force? In doing so, we will also mention Newton's third law of motion. Scroll down to learn how to calculate normal force.
What is normal force?
Normal force is the perpendicular force that a surface exerts on an object. For example, if you place a book on a table, it will be pulled toward the ground by gravitational force. To counteract this force, the table puts pressure on the book, preventing it from falling. This opposing force is called normal force and is denoted FN or N. The unit of normal force is “N” (Newton).
Normal force calculator
Normal force is a typical example of Newton's third law of motion.
If one object exerts a force on a second object, the second object exerts a force of equal magnitude and opposite direction on the first object (action equals reaction).
So, normal force is equal to the force exerted by an object on a surface. Its formulas change depending on the slope of the surface.
For an object lying on a flat surface, the formula is as follows: FN = m * g
Where
m is the mass of the object. g – free fall acceleration. According to Newton's third law, the normal force (FN) for an object on a flat surface is equal to its gravitational force (W).
For an object placed on an inclined surface, the normal force equation is: FN = m * g * cos (α)
Where
α is the angle of inclination of the surface.
On an inclined surface (assuming the object is not sliding down), the object's weight is supported by both normal force and friction. The gravitational force of an object is not opposite and is equal to the normal force.
For objects on a flat surface, the normal force opposes the weight of the objects. (Don't confuse this with mass! Weight is the same thing as gravity.) This is only the case when there is no external force on the object, or, if there is one, the external force is parallel to the surface. Let's see what happens if there is an external force that is not acting at the same time as the surface!
Formula for normal force with external force When calculating with external force, only the parallel component of the vector should be taken into account. This is why angles are included in the normal force equations listed below.
1. External downward force
FN = m * g + F * sin (x)
Where
F – value of external force. x is the angle between the surface and the external force.
2.External upward force
FN = m * g – F * sin (x)
If there is an external downward force, you need to add its vector component to the weight of the object. This increases the normal force, the external force pushes the object into the ground. The opposite is the case for an external force directed upward. It pushes the object away from the ground, so the normal force is reduced.
If the force is straight up and equal to the force of gravity, the normal force is zero. Why? Because it completely counters the force of gravity.
How to find normal strength yourself?
Imagine that there is a box on the ground that you want to move. Weight 100 kg. You press it at a 45 degree angle with a force of 250 N.
N = 100 * 9.807 + 250 * sin (45o) = 980.7 + 250 * √2 / 2 = 1.157.4 N
The earth exerts a force of 1157.4 N on the box.
Resultant force
To obtain the resulting force vector, it is necessary to add up all the force vectors acting on the body.
Everyone knows Ivan Krylov’s fable about the Swan, Cancer and Pike, in which representatives of the animal world tried to move a cart that “is still there.” Let us remember that the swan was pulling the cart “into the clouds,” the crayfish was “backing away,” and the pike was “pulling it into the water.”
If this was the case, then the cart is unlikely to rest in place.
Let us assume that a cart weighing 100 kg is acted upon by the forces indicated in the figure:
Fleb = 150 N; Frak = 125 N; Fpike = 65 N; α = 210°
Let's try to determine the location of the cart in 10 seconds. To do this you need:
- Find the resulting force using the operation of vector addition.
- Determine the acceleration vector using the formula: a = ΣF/m
- Calculate the distance traveled in 10 seconds using the formula S = V0(t1-t0) + 1/2a(t1-t0)2
Let's start the calculations.
I. Looking for the resultant force
Finding the components of the vectors. For the first two forces it is simple (since they are directed along the X and Y axes):
Fleb = (0;150N); Frak = (125H;0)
For pike it’s a little more complicated:
Fpike = (Fschx;Fschy) = (Fsch cosα;Fsch sinα) = (65 cos210°;65 sin210°) = (65 (-0.87);65 (-0.5)) = (-57Н;-33Н)
For the force exerted by the pike, negative values were obtained. This is confirmed by the vector in the diagram - it is directed to the left (negative X values) and down (negative Y values).
Now we add the vectors:
Fl = ( 0;150N) Fr = (125N; 0) Fsh = (-57N;-32N) ————— F = (68N;118N)
We found not only the magnitude of the resulting force, but also its direction !
II. Determining acceleration
a = ΣF/m = (68Н;116Н)/100kg = (0.68m/s2;1.18m/s2)