What is resonance in physics: the essence of the phenomenon, vibrations and frequency

Oscillations and Frequency

The procedure associated with a change in the position of the system near the equilibrium point and repeated over time is called oscillations. A swinging pendulum repeats its movements relative to the normal to the horizontal plane. At the same time, if additional energy is not applied to its movement, its swinging will fade.

The phenomenon of such changes can be classified according to the following parameters:

• according to the mathematical model used in oscillations;
• according to the periodicity structure;
• by the nature of physical properties;
• by type of interaction with environmental conditions.

Attention! All vibrations, regardless of their physical properties, have general laws that can be described by wave phenomena. These patterns are studied by the theory of wave oscillations.

Mechanical vibrations are associated with the transformation of one form of energy into another, wave vibrations are associated with the spatial movement and distribution of energy.

The common parameters for all oscillations are:

• frequency;
• period;
• amplitude.

Frequency is the number of vibrations performed by a body per unit of time. The unit of measurement is hertz (Hz), the graphic designation is f, ʋ. The frequency can be circular - when a point periodically moves around a circle, it is also called cyclic:

ω = 2π*T, (rad/s).

Period (T) is the time of a whole (complete) oscillation, during which it is possible to record the repetition of any of the characteristics of the system state. This means that she has completed a complete swing. The designation of the period is T, the unit of measurement is second (s).

The two quantities T and f are reciprocals, as follows from the formulas:

• T = 1/f;
• f = 1/T.

The greatest deviation of a point of a body or any quantity of a system from an equilibrium position is called the amplitude of oscillations and is denoted by the letter A. The unit of measurement is those quantities whose changes are being considered. For mechanical deviations, the amplitude is measured in meters (m), the amplitude of alternating voltage is measured in volts (V), and so on.

Period and frequency of mechanical vibrations

What is EPR

Electron paramagnetic resonance (EPR) spectroscopy is widely used in studies of physicochemical and biological phenomena. EPR makes it possible to study the mechanisms of various bioenergetic processes associated with the transfer of electrons and energy, biological damage during irradiation, and solve some structural problems. It is not possible to even briefly mention all areas of application of EPR, since this is one of the universal spectral methods.

A necessary condition for recording electron paramagnetic resonance is the presence of the molecules under study with their own magnetic moment, which consists of the spin and orbital components. This means that such (paramagnetic) molecules must contain one or more unpaired electrons. Placing a paramagnetic molecule in a constant magnetic field causes the appearance of additional energy levels, transitions between which, as in optical spectroscopy, can be caused by the application of a second - electromagnetic - field.

What is EPR.

The EPR method measures the power absorption of this electromagnetic field in a sample. Registration of changes in absorption depending on the magnitude of the applied magnetic field results in an ESR spectrum characteristic of the molecules under study, their dynamics and interactions.

The proposed task involves preparing samples and independently measuring EPR signals of solutions containing so-called molecules. spin labels (in this case, stearic acid with a paramagnetic group attached to it). Spin markers are widely used in various molecular biological studies, in particular for measuring microviscosity, since the shape of their EPR spectra changes depending on the viscosity of the molecular environment. The task will involve measuring the spectra of spin labels under conditions of different environmental viscosities and subsequent simple calculations using a modern research spectrometer EMX-6 (Brooker, Germany).

Interesting material: What is electrolysis and how is it used in industry.

What information can you get from EPR?

Only the electron paramagnetic resonance or EPR method clearly detects unpaired electrons. Other methods, such as fluorescence, can provide indirect evidence of the presence of free radicals, but only ESR alone provides conclusive evidence of their presence. In addition, EPR has a unique ability to identify detected paramagnetic centers. EPR samples are very sensitive to their environment, so the technique sheds light on the molecular structure near the unpaired electron.

Sometimes EPR spectra show strong changes in line shapes, giving insight into dynamic processes such as molecular motion or fluidity. The EPR spin trap technique, which detects short-lived reactive free radicals, illustrates very well how radical detection and identification can be used. This method is vital in the biomedical field for elucidating the role of free radicals in many pathologies and determining toxicity.

ESR spin labeling is a technique used by biochemists in which a paramagnetic molecule (spin label) is used to “tag” macromolecules in specific areas. From the EPR spectra reported by the spin label, it is possible to determine the type of medium (its hydrophobicity, pH, fluidity and other parameters) in which the spin label is located.

ESEEM and ENDOR are two EPR methods that measure the interaction of an electron with surrounding nuclei. They are extremely powerful methods for studying the structure of "active sites" in metalloproteins. Other important applications for quantitative ESR are radiation dosimetry, dose measurements for sterilization of medical products and food, detection of irradiated foods, and dating of early human artifacts.

How does EPR work?

EPR is a magnetic resonance technique very similar to NMR (nuclear magnetic resonance). However, instead of measuring nuclear transitions in our sample, we detect transitions of unpaired electrons in an applied magnetic field. Like a proton, an electron has a "spin", which gives it a magnetic property known as a magnetic moment. The magnetic moment causes the electron to behave like a tiny bar magnet, like the one you might put on your refrigerator.

It will be interesting➡ All about Ohm’s law: in simple words with examples for dummies

When we apply an external magnetic field, the paramagnetic electrons can either align in a direction parallel or antiparallel to the direction of the magnetic field. This creates two different energy levels for the unpaired electrons and allows them to be measured as they move between the two levels. Initially, there will be more electrons at the lower energy level (i.e., parallel to the field) than at the higher level (antiparallel). We use a fixed frequency of microwave radiation to excite and move some electrons in the lower energy level to the upper energy level.

In order for this transition to occur, we must also apply an external magnetic field with a certain strength (induction), so that the energy level separation between the lower and upper states exactly corresponds to our microwave frequency. In fact, to achieve this condition, we “stretch” the field of an external magnet by placing the sample under study inside the spectrometer at a fixed frequency of microwave radiation. The condition where the magnetic field and microwave frequency are matched to create resonance (or absorb microwave energy) is known as the resonance condition.

Nuclear magnetic resonance.

The essence of the phenomenon of electron paramagnetic resonance is the resonant absorption of electromagnetic radiation by unpaired electrons. The presence of a spin moment in a negatively charged electron leads to the appearance of an electronic magnetic moment µe, which is proportional to the spin S and is determined by the expression:

µe = gβS, (1)

where g is a dimensionless constant (the so-called electron g-factor) is the ratio of the magnetic moment of an electron to its mechanical moment, equal to 2.002 for a free electron, β is Bohr’s electron magneton, β = 9.27400915(26) 10-24 J/T .

The interaction energy between the electron magnetic moment and the external magnetic field is described by the following expression:

Evz =-µeB =gβBSB, (2)

where SB is the projection of the spin onto the direction of the magnetic field. Let's consider the case with one unpaired electron. When a constant external magnetic field is applied in accordance with the Zeeman effect, two levels with magnetic quantum numbers ms=±½ will appear with splitting ∆E=gβH between them. The magnitude of splitting is directly proportional to the intensity of the applied magnetic field and in absolute value is 100-1000 times less than the energy of thermal motion kT. Mathematically, the ratio of the populations of levels with ms=+½ and ms=-½, according to the Boltzmann distribution, is expressed by the following formula:

N=1/2/N-1/2 = e-∆E/kT = e-gβH/kT (3)

If an electron placed in a constant magnetic field is exposed to electromagnetic radiation of the microwave range with the plane of polarization of the magnetic field B1 perpendicular to the plane of the constant field, then if the condition is met:

hν = gβH (4)

resonant transitions between two levels are induced, at which the electron changes its spin state (in other words, the spin flips). Since the levels differ in population, this effect will be expressed in total in the form of absorption of electromagnetic field energy by the system. The main objective of the experiment when observing the ESR phenomenon is the accurate registration of absorbed electromagnetic energy.

Use of EPR in medicine.

Structure and properties of EPR spectra

The behavior of magnetic moments in a magnetic field depends on various interactions of unpaired electrons, both among themselves and with their immediate environment. The most important of them are spin-spin and spin-orbit interactions, interactions between unpaired electrons and the nuclei on which they are localized (hyperfine interactions), interactions with the electrostatic potential created by ions in the immediate environment at the location of unpaired electrons, and others. Most of the listed interactions lead to a natural splitting of lines. In the general case, the EPR spectrum of a paramagnetic center is multicomponent.

The main characteristics of the EPR spectrum of a paramagnetic center (PC) are:

• the number of lines in the EPR spectrum of a particular PC and their relative intensities.
• Fine structure (FS). The number of TC lines is determined by the spin value S of the PC and the local symmetry of the electrostatic field of the immediate environment, and the relative integral intensities are determined by the quantum number mS (the magnitude of the projection of the spin onto the direction of the magnetic field). In crystals, the distance between the TC lines depends on the magnitude of the crystal field potential and its symmetry.
• Ultrafine structure (HFS). HFS lines from a particular isotope have approximately the same integral intensity and are practically equidistant. If the PC core has several isotopes, then each isotope produces its own set of HFS lines. Their number is determined by the spin I of the isotope nucleus, around which the unpaired electron is localized. The relative intensities of the HFS lines from different PC isotopes are proportional to the natural abundance of these isotopes in the sample, and the distance between the HFS lines depends on the magnetic moment of the nucleus of a particular isotope, the hyperfine interaction constant, and the degree of delocalization of unpaired electrons on this nucleus.

Super ultrafine structure (USHS). The number of CCTS lines depends on the number nl of equivalent ligands with which the unpaired spin density interacts and the value of the nuclear spin Il of their isotopes. A characteristic feature of such lines is also the distribution of their integral intensities, which in the case of Il = 1/2 obeys the law of binomial distribution with the exponent nl. The distance between the SCHS lines depends on the magnitude of the magnetic moment of the nuclei, the hyperfine interaction constant and the degree of localization of unpaired electrons on these nuclei.

Spectroscopic characteristics of the line

A special feature of EPR spectra is the form in which they are recorded. For many reasons, the EPR spectrum is recorded not in the form of absorption lines, but as a derivative of these lines. Therefore, in EPR spectroscopy, a slightly different terminology, different from the generally accepted one, is adopted to designate line parameters.

EPR absorption line and its first derivative: 1 – Gaussian shape; 2 – Lorentzian form.

• The true line is a δ-function, but taking into account relaxation processes it has a Lorentz form.
• Line – reflects the probability of the process of resonant absorption of electromagnetic radiation by the PC and is determined by the processes in which spins participate.
• The shape of the line reflects the law of probability distribution of resonant transitions. Since, to a first approximation, deviations from resonant conditions are random, the shape of lines in magnetically diluted matrices has a Gaussian shape. The presence of additional exchange spin-spin interactions leads to a Lorentzian line shape. In general, the shape of a line is described by a mixed law.
• The line width – ΔВmax – corresponds to the distance across the field between the extrema on the curved line.
• The line amplitude – Imax – corresponds on the signal amplitude scale to the distance between the extrema on the curved line.
• Intensity – I0 – probability value at the MAX point on the absorption curve, calculated by integration along the contour of the recording line;
• Integrated intensity - the area under the absorption curve, is proportional to the number of paramagnetic centers in the sample and is calculated by double integration of the recording line, first along the contour, then over the field.
• The position of the line – B0 – corresponds to the intersection of the contour of the derivative dI/dB with the zero line (trend line).
• position of EPR lines in the spectrum.

It will be interesting➡ What is an electric field: an explanation in simple words

According to the expression ħν = gβB, which determines the conditions of resonant absorption for a PC with spin S = 1/2, the position of the electron paramagnetic resonance line can be characterized by the value of the g-factor (analogue of the Lande spectroscopic splitting factor). The value of the g-factor is defined as the ratio of the frequency ν at which the spectrum was measured to the value of magnetic induction B at which the maximum effect was observed. It should be noted that for paramagnetic centers the g-factor characterizes the PC as a whole, i.e., not a separate line in the EPR spectrum, but the entire set of lines caused by the PC under study.

The essence of the phenomenon of resonance

Resonance - what is it?

The word resono is Latin for response. An oscillating system responds to external oscillatory influences. As the external frequency approaches its own frequency, it responds with a sharp increase in the amplitude of its forced periodic deviations from the equilibrium state.

Resonance phenomenon

Important! Resonance and unison are not the same phenomena. Unison is the coincidence of sounds in tone. In this case, there is no increase in the amplitude of sound vibrations, but a “one-voice” of two or more sound sources occurs.

Two strings can sound in unison if a force is simultaneously applied to them, causing them to vibrate. But one can resonate with the other at the moment their vibration frequencies coincide and increase the volume of its sound.

Types of resonance

There are a large number of types of resonance in physics. They are all similar in some ways and different in some ways, namely in their characteristics and the nature of their appearance. Among them are:

• mechanical and acoustic resonances;
• electric;
• optic;
• orbital fluctuations;
• atomic, particle and molecular.

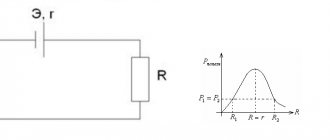
Process graph in an oscillatory circuit

The following subsections will describe each of these types in more detail.

Mechanical and acoustic

The most popular and obvious mechanical type would be the resonant swing, which was mentioned earlier. If you push them at certain moments, taking into account their frequency, the scope of their movement will increase or die out if no force is applied.

Mechanical resonators are based on the conversion of potential energy into kinetic energy and vice versa. If we consider a pendulum, then all its energy is potential at rest. It converts to kinetic when he passes the bottom point at his maximum speed.

Devices for organizing resonance

Important! Some mechanical systems are capable of storing potential energy and using it in various forms. An example is a spring that stores compression, which is the binding energy of atoms

The acoustic type of resonance can be found in some musical instruments such as guitar, violin, and piano. They have a fundamental resonant frequency that depends on the length, mass and tension of the strings.

Acoustic resonance helps people find defects in pipelines

In addition to the fundamental frequency, the strings of these musical instruments have resonance at higher harmonic vibrations of the fundamental frequency. If a string is pulled, it will begin to vibrate at all frequencies that are inherent in a given impulse, but frequencies that do not coincide with the resonance will very quickly die out, and the human ear will hear only harmonic vibrations, which are notes.

Acoustic systems, microphones and loudspeakers do not tolerate resonance of individual parts of their housing, as this reduces the uniformity of their amplitude-frequency characteristics and degrades the quality of sound reproduction.

Strings create acoustic resonance

Resonance electrical

There is also resonance in electronics. It refers to the state or mode of a passive electrical circuit containing coils and capacitors, in which its input reactance and conductivity will be zero. This means that at resonance, the current at the input to the circuit, if any, will be in phase with the voltage.

Oscillatory circuit

In electricity, resonance is achieved when induction and reaction capacitance are balanced. This equality allows energy to circulate between the inductive elements and their magnetic field, and the electric field in the capacitor.

The resonance mechanism itself is based on the fact that the MF of the inductance creates an electric current, which charges the capacitor, discharging it and creating this magnetic field. The simplest device based on this interaction is an oscillatory circuit capable of producing resonance of voltages and currents.

Light optical resonance model

Optical resonance

And there is resonance in the optical range. One of its most popular examples is the Fabry-Perot resonator. It is formed by several mirrors, between which a so-called resonating standing wave is established. In addition, ring resonating systems with a traveling wave and microscopic resonators with standing waves are used.

Oscillatory circuit diagram

Orbital wobble

Oscillations in astrophysics are situations where there are two or more celestial objects that have certain orbital periods that correlate like small natural numbers. As a result of this effect, celestial objects exert a constant gravitational attraction on each other. It stabilizes their orbits.

There are also fluctuations in the orbits of celestial bodies.

The benefits and harms of mechanical resonances

When constructing tasks and engineering structures, a mandatory condition is to check structures for resonance phenomena. At the same time, all sources of vibrations are studied, both natural (wind, surf) and artificial (radars, transmitting antennas).

Resonance in an electrical circuit

One example of the harm of resonance is the destruction of a suspension bridge in Washington state, USA, in 1940. The low height of the supports of the Tacoma structure caused involuntary vibrations when exposed to wind. As a result of the fact that these vibrations once came into resonance with the gusts of movement of air masses, the bridge collapsed. Although the appearance of this phenomenon was noted during construction, it was not given any importance.

The phenomenon of amplitude amplification when frequencies coincide during an earthquake causes destruction and huge tsunami waves.

Resonance has advantages:

• resonators on string instruments enhance harmonics by amplifying standing waves;
• the oscillatory circuit of radio receiving devices, when tuned to a transmitting station, amplifies the received signal in amplitude;
• The destructive features of this process for concrete are used during the operation of a hammer drill, during vibration during drilling.

Interesting. Thanks to the phenomenon under consideration, modern medicine has acquired such an indispensable device as MRI - magnetic resonance imaging. Using MRI, a complete examination of the human body is performed. Magnetic resonance therapy allows you to treat diseases of the musculoskeletal system without surgical intervention.

Tsunami is the result of resonance of the frequency of sea waves with the frequency of tremors

Theoretical and practical significance

Electron paramagnetic resonance (EPR), the resonant absorption of electromagnetic energy in the centimeter or millimeter wavelength range by substances containing paramagnetic particles. EPR is one of the radiospectroscopy methods. Paramagnetic particles can be atoms and molecules, usually with an odd number of electrons (for example, nitrogen and hydrogen atoms, NO molecules); free radicals (for example, CH3); ions with partially filled internal electron shells (for example, nones of transition elements); color centers in crystals; impurity atoms (for example, donors in semiconductors); conduction electrons in metals and semiconductors.

What is EPR.

EPR was discovered by E.K. Zavoisky in 1944. Since 1922, a number of works have expressed considerations about the possibility of the existence of EPR. An attempt to experimentally detect EPR was made in the mid-30s. Dutch physicist K. Gorter and his colleagues. However, ESR could only be observed thanks to radio spectroscopic methods developed by Zavoisky. EPR is a special case of magnetic resonance. Its description within the framework of classical physics is as follows: in an external constant magnetic field H, the magnetic moment vector m precesses around the direction of the magnetic field H with a frequency v determined by the relation

2pv = gH

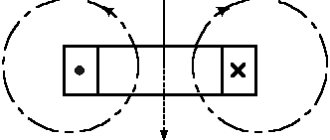
Here g is the gyromagnetic ratio. The precession angle q (the angle between the vectors H and m) remains constant. If the system is placed in a magnetic field H1^H, rotating around H with frequency v, then the projection of the vector m onto the direction of the field H will change with frequency v1 = gH1/2p. This change in the projection m with frequency v1 under the influence of the radio frequency field H1 (Fig. 1) has a resonant nature and determines the ESR. When studying EPR, a linearly polarized alternating magnetic field is usually used, which can be represented as the sum of two fields rotating in opposite directions with a frequency v. One of the components, coinciding in the direction of rotation with precession, causes a change in the projection of the magnetic moment m on H.

The above classical consideration is convenient for analyzing relaxation processes (see below). To describe the EPR spectra, a quantum approach is required. Absorption of electromagnetic energy occurs in the case when the quantum of electromagnetic energy hv (h - Planck's constant) is equal to the energy difference DE between magnetic (Zeeman) sublevels formed as a result of splitting the energy levels of a paramagnetic particle in a constant magnetic field H.

If the magnetic moment of a paramagnetic particle is due only to the electron spin S = 1/2, then m = gsbMs, where gs = 2.0023 is the spectroscopic splitting factor for a free electron, b is the Bohr magneton, and Ms is the magnetic quantum number, taking values ​​± 1 /2. In an external static magnetic field H, these electrons of paramagnetic particles are divided into 2 groups with energies - gsbH/2 and + gsbH/2. Quantum transitions are possible between these groups of levels, which are excited by the field H1^H.

Electronic resonance using the example of atoms.

During the transition from the lower level to the upper one, electromagnetic energy is absorbed, and during the reverse transition it is emitted. The probability of these processes is the same, but since under equilibrium conditions the population of the lower level is greater than that of the upper level, energy is absorbed. If a population inversion is created in some artificial way, then under the influence of an electromagnetic field the system will radiate energy. This principle underlies the operation of paramagnetic quantum amplifiers.

Typically, the paramagnetism of particles is due to the total contribution of the orbital and spin moments of several electrons; Moreover, in crystals these electrons are affected by strong electric fields of surrounding ions (ligands). Therefore, describing the structure of EPR spectra in this case is a difficult task. To calculate the spectra, a semi-empirical method proposed by A. Abraham (France) and H. M. L. Price (USA) in 1951, called the spin Hamiltonian method, is used. During EPR, transitions occur between nearby levels. The calculation of energy levels in a magnetic field is simplified if we introduce the effective spin S, the absolute value of which is determined by the number n of nearby levels: n = 2S + 1.

EPR.

The energies are calculated under the assumption that the magnetic moment of the particle is determined by the value S. Then the level energy E =gbMsH, where Ms takes (2S + 1) values: S, (S - 1), ...... - (S - 1), - S. The value of the g-factor can differ significantly from the value of the g-factor of a free electron gs. Between levels that differ in Ms by DMs = ± 1, dipole transitions are possible, and the resonance conditions will still be described by the formula with gs = g. If S > 1/2, then energy levels with different |Ms| can split at H = 0, and several absorption lines appear in the EPR spectrum (fine structure of the EPR spectrum).

The interaction of electrons with the magnetic moment of the nucleus of a paramagnetic atom leads to the appearance of a hyperfine structure in the EPR spectrum. If the nuclear spin is I, then the number of hyperfine components is equal to 2I + 1, which corresponds to the transition condition DMI = 0, where MI is the nuclear magnetic quantum number (Fig. 3, b). The interaction of the electrons of a paramagnetic particle with the magnetic moments of the nuclei of surrounding ions also splits the EPR line (superhyperfine structure, Fig. 4). The study of hyperfine and superhyperfine interaction makes it possible to determine the location of unpaired electrons.

Material on the topic: What is current power and how to calculate it.

Paramagnetic relaxation. Width of lines. Relaxation processes that restore equilibrium in the system of electron spins, disturbed as a result of the absorption of electromagnetic energy, are characterized by relaxation times T1 and T2. The width of the absorption lines Dv is related to the relaxation times by the relation:

It will be interesting➡ What is grounding in simple words

Dn = (1/ T1) + (1/ T2). (4)

In the classical consideration, the times T1 and T2 are called the longitudinal and transverse relaxation times, since they determine the time for restoration of the equilibrium position of the longitudinal and transverse components of the magnetization vector. Since the restoration of the equilibrium value of the transverse component of magnetization occurs due to the interaction between the magnetic moments of paramagnetic particles (spin-spin interaction), T1 is also called the spin-spin relaxation time.

The restoration of the longitudinal component is due to the interaction of the magnetic moments of paramagnetic particles with vibrations of the crystal lattice (spin-lattice interaction). Therefore, the time T1 is also called the spin-lattice relaxation time. It characterizes the rate of restoration of equilibrium between the spin system and lattice vibrations.

The spin-spin interaction consists of two components: dipole-dipole and exchange interactions. The local field acting on a paramagnetic particle consists of the external field H and the field ND created by dipoles (magnetic moments) of neighboring paramagnetic particles. The ND field changes from point to point, since the set of neighboring paramagnetic particles and the direction of their magnetic moments changes, which leads to a broadening of the EPR line. Exchange interaction, on the contrary, tends to order the directions of spins and, therefore, reduces the “chaotic” orientation of the magnetic moments of paramagnetic particles. Therefore, it leads to “exchange narrowing” of the EPR line.

The movements of the nuclei of paramagnetic centers create electric field fluctuations that affect the orbital motion of electrons, which, in turn, leads to the appearance of local magnetic field fluctuations and, consequently, to the broadening of EPR lines. The magnitude of the spin-lattice interaction decreases with decreasing temperature, since the amplitude of thermal vibrations of the nuclear lattice decreases. The magnitude of the spin-spin interaction is practically independent of temperature. Therefore, for transition metal ions with a large contribution of orbital momentum, the ESR line can be observed only at low temperatures.

ESR spectra are observed at a sufficiently low power of the alternating electromagnetic field (10-2-10-3 W), when the steady state differs little from the equilibrium one. If the power is high and relaxation processes are not able to restore the equilibrium distribution, then the populations of the levels level out and saturation occurs, which is detected by a decrease in absorption. The level saturation effect is used to measure paramagnetic relaxation times. Experimental methods. EPR is observed in the microwave range. The intensity of energy absorption increases with increasing frequency, since in accordance with (3) the difference in the population of levels increases.

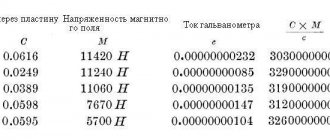
A fairly high sensitivity of the method is achieved at a frequency of v = 9000 MHz. This corresponds to H = 3200 Oe (the magnitude of the magnetic field, easily obtained in laboratory conditions). The use of powerful electromagnets and superconducting solenoids allows operation at frequencies up to n = 150,000 MHz (wavelength l = 2 mm).

To measure absorption, radio spectrometers (EPR spectrometers) are used, in which, at a constant frequency and a slow change in the external magnetic field, the change in power absorbed in the sample is recorded. In direct amplification EPR spectrometers, high-frequency oscillations from a klystron are fed through a waveguide path into a volumetric resonator (a cavity of ~ l size) placed between the poles of an electromagnet.

What is a cavity resonator?

Electromagnetic waves passing through the resonator or reflected from it enter the crystal detector. The change in power absorbed in the sample is recorded by the change in the detector current. To increase the sensitivity of the spectrometer, the external magnetic field is modulated with a frequency of 30 Hz - 1 MHz. If there is absorption in the sample, microwave waves transmitted or reflected from the resonator also turn out to be modulated. The modulated signal is amplified, detected and fed to a recording device (oscilloscope or recorder).

In this case, the recorded signal has the form of a derivative of the absorption curve. The sensitivity of an EPR spectrometer is determined by the level of thermal noise of the amplifier. In superheterodyne spectrometers, power is supplied to the detector from an additional klystron. The frequency of oscillations generated by this klystron is different from the frequency of the signal klystron. The signal from the detector is amplified at a difference frequency of 30-100 MHz.

The EPR spectra of transition metal ions are the most well studied. In order to eliminate the line broadening caused by the dipole interaction with neighboring paramagnetic ions, measurements are carried out on single crystals, which are diamagnetic dielectrics, into which paramagnetic ions are introduced as impurities (0.001%-0.1%). The influence of surrounding ions on a paramagnetic ion is considered as the action of point electric charges. EPR is observed at the populated lower energy levels of a paramagnetic ion, resulting from splitting the main level by the electric field of surrounding charges.

EPR of transition metal ions.

In the case of rare earth ions, the crystal field turns out to be weak compared to the interaction of the electrons of the ion, since the paramagnetism of these ions is due to deep-lying 4 f electrons. The angular momentum of an ion is determined by the sum of the orbital and spin moments of the ground level. In a crystal field, levels with different absolute values ​​of the projection of the total magnetic moment are not equivalent in energy.

For Fe group ions, the paramagnetism of which is due to 3 d-electrons, the crystal field turns out to be stronger than the spin-orbit interaction, which determines the energy spectrum of the free ion. As a result, the maximum value of the orbital momentum projection either decreases or becomes equal to zero. It is commonly said that a partial or complete “freezing” of the orbital momentum occurs.

Quality factor of the oscillatory system

Another characteristic of an oscillatory system (OS) is the quality factor. It is denoted by the letter Q and is found using the general formula:

Resonance frequency: formula

Q = ω0*W/Pd = 2πf0W/Pd ,

Where:

• ω0 – circular resonant frequency;
• f0 – resonance frequency;
• W – energy reserve in the CS;
• Pd – power dissipation.

The quality factor determines the ratio of the energy reserve in the CS to the losses during the interval of phase changes by an amount of 1 radian. It shows the width of the resonant band.

Attention! The formula for calculating Q says that in each period the rate of decay of oscillations and the amount of lost energy are lower, the higher the value of the quality factor of the CS.

Quality factor of the oscillatory system

Positive and negative sides of resonance

High-rise masts and towers, skyscrapers, bridges and observation platforms must withstand an increase in the amplitude of their vibrations as a result of external influences.

The resonance phenomenon has advantages:

• resonators on string instruments enhance harmonics by amplifying standing waves;
• The oscillatory circuit of radio receiving devices, when tuned to a transmitting station, amplifies the received signal in amplitude.

The destructive properties of this phenomenon are used when operating a hammer drill - during vibration during drilling, the concrete wall comes into resonance with the working tool, and concrete destruction occurs at the point of use.

Pros and cons of resonance

Types and examples of resonance

Only in physics itself do we distinguish such types of resonance as:

• Mechanical resonance is the same aforementioned swing, the resonance of a bridge from a passing company of soldiers, the resonance of a bell ringing, etc. In a word, resonance caused by mechanical influences.
• Acoustic resonance is the resonance that makes all stringed musical instruments work: guitar, violin, lute, balalaika, banjo, etc. By the way, the body of musical instruments has its own shape for a reason. The sound produced by the string when plucked enters the body and there enters into resonance with the walls, which as a result leads to its amplification. For this reason, the sound quality of the same guitar greatly depends on the material from which it is made and even on the varnish with which it is coated.
• Electrical resonance is the coincidence of the oscillation frequency of the external voltage with the oscillation frequency of the electrical circuit through which the current flows.

In addition to these purely physical resonances, there is also the public resonance that we have already mentioned - a strong public response to some event (usually political or economic), for example, Brexit, Britain, its withdrawal from the European Union caused a wide public resonance in many European countries and especially, of course, in Britain itself.

There is also cognitive resonance - this is a complete coincidence in views and opinions. For example, you met a new person, and he thinks the same way as you, you have absolutely similar views, tastes, preferences, then cognitive resonance takes place. And the opposite phenomenon is cognitive dissonance, when you absolutely disagree with someone or something and absolutely do not accept what is happening. (For example, the author of this article, finding himself in some Ukrainian bureaucratic institution, be it Zheka, BTI or tax office, experiences real cognitive dissonance)).

Electromechanical resonators

The phenomenon of mechanical resonance is an increase in the amplitude of forced oscillatory movements. An electromechanical resonator is a device designed to measure forces of a mechanical nature and its derivatives. In terms of technical design, it is similar to a piezoelectric sensor, but with a higher quality factor. The main elements of such a device are:

• a piezoelectric plate in the shape of a paired tuning fork (parallel identical rods with ends connected to each other);
• electrodes attached to the ends of the piezoelectric component.

To reduce the frequency, a concentrated mass is used, which is connected to the middle parts of the rods using a jumper.

Electromechanical resonator design

The picture below shows the following zones and elements:

• 1 – rods (the cross-section is uniform throughout the entire rod);
• 2 – combined elements;
• 3 – electrode placement area;
• 4 – concentration masses;
• 5 – jumpers;
• 6 – places for fixing the resonator and connecting the circuit for force measurement.

For your information. Electromechanical resonators are parts or devices that combine the properties of mechanical resonation and piezoelectric transformations.

Types of phenomenon

In describing resonance, G. Galileo drew attention to the most essential thing - the ability of a mechanical oscillatory system (heavy pendulum) to accumulate energy, which is supplied from an external source with a certain frequency. Manifestations of resonance have certain characteristics in different systems and therefore different types are distinguished.

Mechanical and acoustic

Mechanical resonance is the tendency of a mechanical system to absorb more energy when its vibration frequency matches the system's natural vibration frequency. This can lead to severe motion fluctuations and even catastrophic failure in unfinished structures, including bridges, buildings, trains and airplanes. When designing facilities, engineers must ensure that the mechanical resonant frequencies of component parts do not match the oscillatory frequencies of motors or other oscillating parts to avoid a phenomenon known as resonant disaster.

Electrical resonance

Occurs in an electrical circuit at a certain resonant frequency when the circuit impedance is minimum in a series circuit or maximum in a parallel circuit. Resonance in circuits is used to transmit and receive wireless communications such as television, cellular, or radio.

Optical resonance

An optical cavity, also called an optical resonator, is a special arrangement of mirrors that forms a standing wave resonator for light waves . Optical cavities are the main component of lasers, surrounding the amplification medium and providing feedback to the laser radiation. They are also used in optical parametric oscillators and some interferometers.

Light confined within the cavity produces standing waves repeatedly for specific resonant frequencies. The resulting standing wave patterns are called "modes". Longitudinal modes differ only in frequency, while transverse modes differ for different frequencies and have different intensity patterns across the beam cross section. Ring resonators and whispering galleries are examples of optical resonators that do not produce standing waves.

Orbital wobble

In space mechanics, an orbital response occurs when two orbiting bodies exert a regular, periodic gravitational influence on each other. This is usually because their orbital periods are related by the ratio of two small integers. Orbital resonances significantly enhance the mutual gravitational influence of bodies. In most cases, this results in an unstable interaction in which the bodies exchange momentum and displacement until resonance no longer exists.

Under some circumstances, a resonant system can be stable and self-correcting to keep bodies in resonance. Examples are the 1:2:4 resonance of Jupiter's moons Ganymede, Europa and Io and the 2:3 resonance between Pluto and Neptune. Unstable resonances with Saturn's inner moons create gaps in Saturn's rings. A special case of 1:1 resonance (between bodies with similar orbital radii) causes large Solar System bodies to clear out the neighborhoods around their orbits, pushing out almost everything else around them.

Atomic, partial and molecular

Nuclear Magnetic Resonance (NMR) is the name given to the physical resonance phenomenon associated with the observation of specific quantum mechanical magnetic properties of an atomic nucleus when an external magnetic field is present. Many scientific methods use NMR phenomena to study molecular physics, crystals, and non-crystalline materials. NMR is also commonly used in modern medical imaging techniques such as magnetic resonance imaging (MRI).

Achieving Resonance Blur

To partially reduce or blur (soften) the resonance, one of the conditions for reducing the amplitude must be met. The effect of depreciation is to:

• reduce the quality factor of the CS;
• remove the coincidence or intersection of the frequency ranges of the CS and the oscillation frequencies of possible third-party disturbances.

There are many devices and design solutions that allow you to do this. The most successful include:

• insertion of conductors with a smaller cross-section into stranded wires of power lines;
• the use of shock absorbers in transport to reduce vibrations while driving;
• use of damper inserts in pipelines operating under high pressure;
• a ban on walking in step when moving across bridges in a column;
• to prevent buildings from swaying and entering into wind resonance, install “blowers” ​​that supply air against the wind;
• supplying current pulses to a non-rigid part during turning.

One of the universal methods designed to blur resonance suggests using two connected elements. For elements, changes in rigidity occur according to two different laws: linear and nonlinear. A coiled spring and a pressed wire are connected together, which is the damping component of the elastic action.

Examples of harmful resonance

It is difficult to say which resonance is more common in our lives: good or harmful to us. History knows a considerable number of terrifying consequences of the resonance phenomenon. Here are the most famous events where an example of resonance can be observed.

1. In France, in the city of Angers, in 1750, a detachment of soldiers walked in step across a chain bridge. When the frequency of their steps coincided with the frequency of free vibrations of the bridge, the range of vibrations (amplitude) increased sharply. There was a resonance, and the chains broke, and the bridge collapsed into the river.
2. There have been cases when in villages a house was destroyed due to a truck driving along the main road.

As you can see, resonance can have very dangerous consequences, which is why engineers should carefully study the properties of construction objects and correctly calculate their vibration frequencies.

Quartz resonators and electromechanical filters

These are the most common resonators that include quartz crystals. The crystal is cut in the shape of a parallelepiped. Electrodes are sprayed onto the resulting plate in a vacuum. The modes of vibration of such an element depend on the following positions:

• type of quartz plate;
• design of electrodes;
• method of connecting electrodes.

The value of the natural frequency of a quartz resonator is influenced by: the shape, dimensions, elastic modulus and density of the piezoelectric element, as well as the features of fastening the part.

The simplest design of a quartz resonator

Electromechanical filters (EMFs) perform stepwise conversion. At the first stage, electrical pulsations are converted into vibrations of a mechanical nature. The second stage filters them, the third returns them to electrical form again.

Attention! The second stage is a mechanical resonator, it works like a filter. It is made from ferrites with magnetostrictive properties, quartz, iron-nickel alloys, piezoceramic elements and other components.

Block diagram of EMF

Examples of resonance

Another example of this manifestation is the acoustic resonance of cathedral pipes when organ music is played. Loud and beautiful sound occurs as a result of this phenomenon. Pipes are used in various diameters and lengths. An instrument such as a flute sounds exclusively using this effect. Children's swings achieve the greatest swing amplitude when there is a resonance of external and internal vibrations.

The use of nanotechnology has discovered the phenomenon of plasma resonance, in which a surface plasmon is excited from the outside by an electromagnetic wave. Regardless of the consequences of resonating different oscillatory systems, its magnitude can be adjusted. Acoustic, electrical, mechanical and other resonators included in the devices expand the range of their applications.

How is resonance determined?

Using electricity and voltage resonance as an example, it can be determined using special devices: a voltmeter or an oscilloscope. To do this, voltage measurements are made while resonating is being adjusted. At maximum voltage, resonance will be achieved. It is important to understand in which particular system resonance is achieved. For example, in a Tesla transformer, the voltage can reach millions of volts, and to configure it, it is enough to bring the probes a short distance to it and change the parameters, depending on the change in voltage. When the tuning is achieved and the voltage is maximum, this will be resonance.

You might be interested in How to determine battery capacity

Device for demonstrating the resonance of pendulums