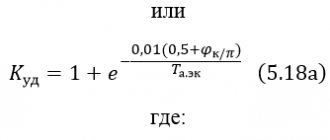What is skin effect?
If you are not strong in English, then skin is translated as skin or, in this case, more like a layer . In Russian-language literature, the skin effect is called the surface effect.
In simple terms, the skin effect is that alternating current flowing through a conductor is forced toward the surface of the conductor with increasing frequency.
The higher the frequency of the signal F, the more it is displaced towards the surface and the thinner the layer through which it flows becomes. This layer is called the skin layer. The red area in the figure is the area through which the signal does not flow.
This leads to the fact that the speed of signal flow at different frequencies is different. This happens because different cross-sectional areas of the conductor are used for different frequencies, and different areas mean different resistance. All this leads to the appearance of phase distortions in the signal.
Properties of fast alternating currents
Definition 1
High-frequency currents are considered to be currents that have a frequency higher than $10,000 Hz$. For these currents the quasi-stationary conditions are not satisfied. As such a current flows through a conductor, eddy currents appear in the conductor, which are generated by changes in the magnetic field at high speed.
Changes in the magnetic field in a conductor occur in such a way that on its axis the eddy current has a direction opposite to the main current, and at the surface of the conductor the flow of this current coincides with the direction of the main current. This means that the high-frequency current has a variable density across the cross section. The current density at the center of the conductor cross-section is almost zero. It increases when moving towards the outer surface. At very high frequencies, current flows through the thin outer layer of the conductor.
Help with student work on the topic High-frequency currents. Skin effect
Coursework 460 ₽ Essay 260 ₽ Test paper 210 ₽
Get completed work or consultation with a specialist on your educational project Find out the cost
Nowadays high frequency currents are widely used. High-frequency melting furnaces are used to quickly heat up metal bodies. Using high-frequency currents, steel parts are hardened. The object is briefly placed inside a coil of high frequency current. The surface layer of the part is heated by eddy currents, while its interior remains cold. The part is removed from the coil, the inner part quickly takes away heat from the surface layer, the surface quickly cools and hardens. The heating depth is controlled by the holding time of the part in the coil and the frequency of the current. After this procedure, the surface of the part becomes hard and durable, while the metal inside retains elasticity and ductility.
What frequency is considered high?
As already mentioned, the skin effect appears only on an alternating signal and only at high frequencies. Before this, I deliberately avoided numerical frequency values. But what does high frequency mean?
Here it is worth focusing on the fact that “high frequencies” mean high by the standards of electronics, and not by human hearing. They begin to combat the manifestation of the skin effect at frequencies above 1 MHz . It can even go so far as to make the conductors not solid, but hollow in the form of tubes. Because the central part of the conductor becomes not only unnecessary, but also harmful to the signal.
Of course, the skin effect also manifests itself in the audible frequency range. It’s not for nothing that all the high-end publications are full of stories about this. But cunning marketers don’t talk about how this influence manifests itself.
Oil Journey
Now the oil has reached the surface, has undergone primary processing and is ready to move on.
There are dozens, or even hundreds of kilometers of pipeline ahead of it, and along this route it is also better to maintain its temperature constantly. This is an issue of environmental safety.
And at the same time, it’s a way to avoid large financial losses if something suddenly happens to the pipeline.
To prevent destruction and downtime, you can build tank farms along the entire length of the pipeline and, in critical situations, drain frozen oil there, but this is very expensive.
Another option is to drain onto the terrain, that is, simply onto the ground. After such drains, nature can take several decades to recover.
But there is a third way to solve the problem - installing special heating systems based on the skin effect on the pipeline. This is exactly the solution offered by the Group’s specialists (SST Group of Companies). This is one of the four global manufacturers of electric heating systems based on skin effect.
Skin effect for specific frequencies
Today there are quite a few online calculators that calculate the thickness of the skin layer for a specific frequency. I liked this one . We will count on it.
Now let's find out the thickness of the skin layer for the maximum audible frequency. It is believed that we hear at best up to 20 kHz. But there is evidence that in the cochlea of the hearing aid there are special hairs immersed in the lymph that sense frequencies up to 100 kHz. These frequencies, although we cannot hear them, affect the perception of the audible range...
It doesn’t matter) in general, for 100 kHz the thickness of the skin layer is 0.2 mm .
If you take a wire with a radius equal to the thickness of the skin layer, then you can’t give a damn about the skin effect. Because its thickness is the entire wire.
You can see the thickness of the wires used in headphones by cutting one of your headphones or, for example, in the article “how to fix headphones without a soldering iron.” Today it is almost standard to make such wires from Litz wire.
Litz wire is a stranded wire, each core of which has a separate varnish insulation.
So the thickness of each wire is much less than the result obtained. This is how the pages of beautiful magazines beautifully deceive us.
Skin layer
Physical picture of occurrence
The mechanism of the skin effect.
An alternating current in a conductor generates an alternating eddy magnetic field, the lines of force of which are perpendicular to the axis of the conductor. Due to electromagnetic induction, an alternating magnetic field generates an eddy electric field, causing the flow of a Foucault eddy current, and on the surface of the conductor the eddy current is directed in the direction of the conductor current, and inside the conductor - opposite. This phenomenon reduces the current in the core of the conductor and increases the surface current. Consider a cylindrical conductor through which current flows. There is a magnetic field around a conductor carrying current, the lines of force of which are concentric circles centered on the axis of the conductor. As a result of an increase in current strength, the magnetic field induction increases, but the shape of the field lines remains the same. Therefore, at each point inside the conductor, the derivative ∂ B ∂ t {\displaystyle {\frac {\partial \mathbf {B} }{\partial t}}} is directed tangent to the magnetic field induction line and, therefore, the line ∂ B ∂ t {\displaystyle {\frac {\partial \mathbf {B} }{\partial t}}} are also circles coinciding with the lines of magnetic field induction. Changing magnetic field according to the law of electromagnetic induction:
rot E = − ∂ B ∂ t {\displaystyle \operatorname {rot} \,\mathbf {E} =-{\frac {\partial \mathbf {B} }{\partial t}}}
creates an electric induction field, the lines of force of which represent closed curves around the line of induction of the magnetic field. The vector of the induction field strength in areas closer to the axis of the conductor is directed opposite to the vector of the electric field strength creating the current, and in areas further away it coincides with it. As a result, the current density decreases in the paraxial regions and increases near the surface of the conductor, that is, a skin effect occurs.
Equation describing the skin effect
We start from Maxwell's equation:
rot B = μ j {\displaystyle \operatorname {rot} \mathbf {B} =\mu \mathbf {j} }
and expressions for j {\displaystyle \mathbf {j} } according to Ohm's law:
j = γ E . {\displaystyle \mathbf {j} =\gamma \mathbf {E} .}
Differentiating both sides of the resulting equation with respect to time, we find:
rot ∂ B ∂ t = μ γ ∂ E ∂ t , {\displaystyle \operatorname {rot} {\frac {\partial \mathbf {B} }{\partial t}}=\mu \gamma {\frac {\ partial \mathbf {E} }{\partial t}},} − rot rot E = μ γ ∂ E ∂ t , {\displaystyle -\operatorname {rot} \operatorname {rot} \mathbf {E} =\ mu \gamma {\frac {\partial \mathbf {E} }{\partial t}},}
here γ {\displaystyle \gamma } is the specific conductivity of the conductor material, γ = 1 / ρ , {\displaystyle \gamma =1/\rho ,\ \ } ρ {\displaystyle \rho } is the specific resistance of the conductor material.
Since rot rot E = grad div E − ∇ 2 E {\displaystyle \operatorname {rot} \operatorname {rot} \mathbf {E} =\operatorname {grad} \operatorname {div} \mathbf {E} -\nabla ^{2}\mathbf {E} } and div E = 0 {\displaystyle \operatorname {div} \mathbf {E} =0} we finally get:
∇ 2 E = μ γ ∂ E ∂ t {\displaystyle \nabla ^{2}\mathbf {E} =\mu \gamma {\frac {\partial \mathbf {E} }{\partial t}}} .
here μ {\displaystyle \mu } is the absolute magnetic permeability of the conductor material, μ = μ 0 μ m , {\displaystyle \mu =\mu _{0}\mu _{m},\ \ } μ 0 {\displaystyle \ mu _{0}} is the magnetic permeability of vacuum, μ m {\displaystyle \mu _{m}} is the relative magnetic permeability of the conductor material.
Skin effect in an infinite conductor with a flat boundary.
To simplify the solution, we assume that the current flows along the X axis {\displaystyle X} along a homogeneous infinite conductor occupying the half-space y > 0 {\displaystyle y>0} . The surface of the conductor is the plane Y = 0. {\displaystyle Y=0.} Thus:
jx = jx ( y , t ) , jy = jz = 0 , {\displaystyle j_{x}=j_{x}(y,t),\qquad j_{y}=j_{z}=0,} E x = E x ( y , t ) , E y = E z = 0. {\displaystyle E_{x}=E_{x}(y,t),\qquad E_{y}=E_{z}=0.}
Then:
∂ 2 E x ∂ y 2 = μ γ ∂ E x ∂ t . {\displaystyle {\frac {\partial ^{2}E_{x}}{\partial y^{2}}}=\mu \gamma {\frac {\partial E_{x}}{\partial t}} .}
In this equation, all quantities depend harmoniously on t, {\displaystyle t,} and we can put:
E x ( y , t ) = E 0 ( y ) ei ω t , {\displaystyle E_{x}(y,t)=E_{0}(y)e^{i\omega t},}
here ω {\displaystyle \omega } is the angular frequency.
Let's substitute this into our equation and get the equation for E 0 ( y ) : {\displaystyle E_{0}(y):}
∂ 2 E 0 ∂ y 2 = i γ μ ω E 0 . {\displaystyle {\frac {\partial ^{2}E_{0}}{\partial y^{2}}}=i\gamma \mu \omega E_{0}.}
The general solution to this equation is:
E 0 = A 1 e − ky + A 2 eky . {\displaystyle E_{0}=A_{1}e^{-ky}+A_{2}e^{ky}.}
Considering that k = i γ μ ω = α ( 1 + i ) {\displaystyle k={\sqrt {i\gamma \mu \omega }}=\alpha (1+i)} , where α = γ μ ω 2 {\displaystyle \alpha ={\sqrt {\frac {\gamma \mu \omega }{2}}}} , we find:
E 0 = A 1 e − α ye − i α y + A 2 e α yei α y . {\displaystyle E_{0}=A_{1}e^{-\alpha y}e^{-i\alpha y}+A_{2}e^{\alpha y}e^{i\alpha y}. }
As we move away from the surface of the conductor ( y → ∞ {\displaystyle y\rightarrow \infty }) the second term increases without limit, which is a physically unacceptable situation. Consequently, A 2 = 0 {\displaystyle A_{2}=0} and only the first term remains as a physically acceptable solution. Then the solution to the problem has the form:
E x = A 1 e − α yei ( ω t − α y ) . {\displaystyle E_{x}=A_{1}e^{-\alpha y}e^{i(\omega t-\alpha y)}.}
Taking the real part of this expression and using the relation j = γ E {\displaystyle \mathbf {j} =\gamma \mathbf {E} } to the current density, we obtain:
jx ( y , t ) = A 1 e − α y cos ( ω t − α y ) . {\displaystyle j_{x}(y,t)=A_{1}e^{-\alpha y}\cos {(\omega t-\alpha y)}.}
Taking into account that jx ( 0 , 0 ) = j 0 {\displaystyle j_{x}(0,0)=j_{0}} is the amplitude of the current density on the surface of the conductor, we arrive at the following distribution of the volumetric current density in the conductor:
jx ( y , t ) = j 0 e − α y cos ( ω t − α y ) . {\displaystyle j_{x}(y,t)=j_{0}e^{-\alpha y}\cos {(\omega t-\alpha y)}.}
Taking into account the effect in technology and combating it[edit | edit code]
The skin effect manifests itself more significantly with increasing frequency of alternating current, and is taken into account when designing and calculating electrical circuits operating on alternating and pulsed current. Since high-frequency current flows through a thin surface layer of the conductor, the total active resistance of the conductor increases, which leads to rapid attenuation of high-frequency oscillations.
The skin effect affects the characteristics of inductors and oscillating circuits, such as quality factor, attenuation in transmission lines, filter characteristics, calculations of thermal losses and efficiency, and the choice of conductor cross-sections.
To reduce the influence of the skin effect, conductors of various sections are used: flat (in the form of tapes), tubular (hollow inside), and a layer of metal with a lower resistivity is applied to the surface of the conductor. For example, silver has the highest conductivity among all metals and is easy to apply to metal surfaces, and its thin layer, in which most of the current flows due to the skin effect, has a noticeable decrease (up to 10%) in the active resistance of the conductor. In addition, the sulfide layer formed on the surface of silver does not conduct current and does not participate in the skin effect, in contrast to the oxide-nitrous oxide layer on the surface of copper, which has noticeable conductivity and has semiconductor properties and introduces additional losses at high frequencies.
Silver coating is also used in microwave equipment that uses specially shaped oscillatory circuits: volumetric resonators and specific transmission lines - waveguides
In addition, at such frequencies, attention is paid to reducing surface roughness in order to reduce the length of the current flow path
Gold plating, which does not have an oxide layer, is also used. On the contrary, coating with nickel, tin or tin-lead solder can significantly, several times, increase the resistance of copper conductors at high frequencies.
Thus, in HF equipment they use inductors wound from silver-plated wire; printed and wire conductors, screen surfaces and capacitor plates are often silver-plated. In high-voltage power lines, a copper or aluminum sheathed wire with a steel core is sometimes used [ source?
], in high-power alternators the winding is made of tubes through which distilled water circulates for cooling.
Also, in order to reduce the skin effect, a system of several intertwined and insulated wires is used - Litz winding wire.
When transmitting large powers over long distances, direct current power lines are used - HVDC. Direct current does not cause a skin effect.